Тригонометрические функции

Графики тригонометрических функций: синуса, косинуса, тангенса, котангенса, секанса, косеканса
Тригонометри́ческие фу́нкции — элементарные функции[1], которые исторически возникли при рассмотрении прямоугольных треугольников и выражали зависимости длин сторон этих треугольников от острых углов при гипотенузе (или, что равнозначно, зависимость хорд и высот от центрального угла дуги в круге). Эти функции нашли широкое применение в самых разных областях науки. По мере развития математики определение тригонометрических функций было расширено, в современном понимании их аргументом может быть произвольное вещественное или комплексное число.
Раздел математики, изучающий свойства тригонометрических функций, называется тригонометрией.
К тригонометрическим функциям традиционно причисляют:
- прямые тригонометрические функции:
- синус ([math]\displaystyle{ \sin x }[/math]);
- косинус ([math]\displaystyle{ \cos x }[/math]);
- производные тригонометрические функции:
- тангенс [math]\displaystyle{ \left(\mathrm{tg}\, x=\frac{\sin x}{\cos x}\right) }[/math];
- котангенс [math]\displaystyle{ \left(\mathrm{ctg}\, x = \frac{\cos x}{\sin x}\right) }[/math];
- секанс [math]\displaystyle{ \left(\sec x=\frac{1}{\cos x}\right) }[/math];
- косеканс [math]\displaystyle{ \left(\mathrm{cosec}\, x=\frac{1}{\sin x}\right) }[/math];
- арксинус, арккосинус и т. д.
В типографике литературы на разных языках сокращённое обозначение тригонометрических функций различно, например, в англоязычной литературе тангенс, котангенс и косеканс обозначаются [math]\displaystyle{ \tan x }[/math], [math]\displaystyle{ \cot x }[/math], [math]\displaystyle{ \csc x }[/math]. До Второй мировой войны в Германии и во Франции эти функции обозначались так же, как принято в русскоязычных текстах[2], но потом в литературе на языках этих стран был принят англоязычный вариант записи тригонометрических функций.
Кроме этих шести широко известных тригонометрических функций, иногда в литературе используются некоторые редко используемые тригонометрические функции (версинус и т. д.).
Синус и косинус вещественного аргумента представляют собой периодические, непрерывные и бесконечно дифференцируемые вещественнозначные функции. Остальные четыре функции на вещественной оси также вещественнозначны, периодичны и бесконечно дифференцируемы, за исключением счётного числа разрывов второго рода: у тангенса и секанса в точках [math]\displaystyle{ \pm \pi n + \frac{\pi}{2} }[/math], а у котангенса и косеканса — в точках [math]\displaystyle{ \pm \pi n }[/math].
Графики тригонометрических функций показаны на рис. 1.
Способы определения
Определение для острых углов

Тригонометрические функции острого угла

В геометрии тригонометрические функции острого угла определяются отношениями сторон прямоугольного треугольника[3]. Пусть [math]\displaystyle{ \triangle AOB }[/math] — прямоугольный, с острым углом [math]\displaystyle{ \angle AOB = \alpha }[/math] и гипотенузой [math]\displaystyle{ OB }[/math]. Тогда:
- [math]\displaystyle{ \sin\alpha=\frac{AB}{OB} }[/math] (синусом угла [math]\displaystyle{ \alpha }[/math] называется отношение противолежащего катета к гипотенузе);
- [math]\displaystyle{ \cos\alpha=\frac{OA}{OB} }[/math] (косинусом угла [math]\displaystyle{ \alpha }[/math] называется отношение прилежащего катета к гипотенузе);
- [math]\displaystyle{ \mathrm{tg}\,\alpha=\frac{AB}{OA} }[/math] (тангенсом угла [math]\displaystyle{ \alpha }[/math] называется отношение противолежащего катета к прилежащему);
- [math]\displaystyle{ \mathrm{ctg}\,\alpha=\frac{OA}{AB} }[/math] (котангенсом угла [math]\displaystyle{ \alpha }[/math] называется отношение прилежащего катета к противолежащему);
- [math]\displaystyle{ \mathrm{sec}\,\alpha=\frac{OB}{OA} }[/math] (секансом угла [math]\displaystyle{ \alpha }[/math] называется отношение гипотенузы к прилежащему катету);
- [math]\displaystyle{ \mathrm{cosec}\,\alpha=\frac{OB}{AB} }[/math] (косекансом угла [math]\displaystyle{ \alpha }[/math] называется отношение гипотенузы к противолежащему катету).
Данное определение имеет некоторое методическое преимущество, так как не требует введения понятия системы координат, но также и такой крупный недостаток, что невозможно определить тригонометрические функции даже для тупых углов, которые необходимо знать при решении элементарных задач о тупоугольных треугольниках. (См.: теорема синусов, теорема косинусов).
Определение для любых углов
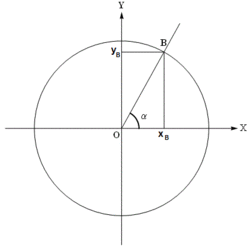
Определение тригонометрических функций
Обычно тригонометрические функции определяются геометрически[4]. В декартовой системе координат на плоскости построим окружность единичного радиуса ([math]\displaystyle{ R=1 }[/math]) с центром в начале координат [math]\displaystyle{ O }[/math]. Всякий угол станем рассматривать как поворот от положительного направления оси абсцисс до некоторого луча [math]\displaystyle{ OB }[/math] (точку [math]\displaystyle{ B }[/math] выбираем на окружности), при этом направление поворота против часовой стрелки считаем положительным, а по часовой стрелке — отрицательным. Абсциссу точки [math]\displaystyle{ B }[/math] обозначим [math]\displaystyle{ x_B }[/math], а ординату — [math]\displaystyle{ y_B }[/math] (см. рисунок 2).
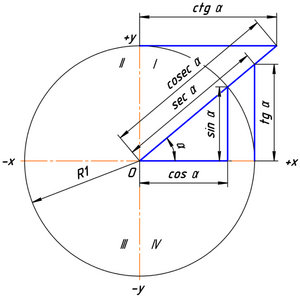
Численные значения тригонометрических функций угла [math]\displaystyle{ \alpha }[/math] в тригонометрической окружности с радиусом, равным единице
Определим функции следующим образом:
- [math]\displaystyle{ \sin\alpha = y_B }[/math], [math]\displaystyle{ \cos\alpha = x_B }[/math];
- [math]\displaystyle{ \operatorname{tg} \alpha=\frac{y_B}{x_B} }[/math], [math]\displaystyle{ \operatorname{ctg} \alpha=\frac{x_B}{y_B} }[/math];
- [math]\displaystyle{ \sec \alpha=\frac{1}{x_B} }[/math], [math]\displaystyle{ \operatorname{cosec} \alpha=\frac{1}{y_B} }[/math].
Нетрудно видеть, что такое определение так же основывается на отношениях прямоугольного треугольника, с тем отличием, что учитывается знак ([math]\displaystyle{ \pm 1 }[/math]). Поэтому тригонометрические функции можно определить и по окружности произвольного радиуса [math]\displaystyle{ R }[/math], однако формулы придётся нормировать. На рисунке 3 показаны величины тригонометрических функций для единичной окружности.
В тригонометрии удобным оказывается вести счёт углов не в градусной мере, а в радианной. Так, угол в [math]\displaystyle{ 360^\circ }[/math] запишется длиной единичной окружности [math]\displaystyle{ 2\pi }[/math]. Угол в [math]\displaystyle{ 180^\circ }[/math] равен, соответственно [math]\displaystyle{ \pi }[/math] и так далее. Заметим, что угол на [math]\displaystyle{ 2\pi }[/math] отличающийся от [math]\displaystyle{ \alpha }[/math] по рисунку эквивалентен [math]\displaystyle{ \alpha }[/math], вследствие чего заключим, что тригонометрические функции периодичны.
Наконец, определим тригонометрические функции вещественного числа [math]\displaystyle{ x }[/math] тригонометрическими функциями угла, радианная мера которого равна [math]\displaystyle{ x }[/math].
Определение как решений дифференциальных уравнений
Синус и косинус можно определить как единственные функции, вторые производные которых равны самим функциям, взятым со знаком минус:
- [math]\displaystyle{ \ \left(\cos x\right)'' = - \cos x, }[/math]
- [math]\displaystyle{ \ \left(\sin x\right)'' = - \sin x. }[/math]
То есть задать их как чётное (косинус) и нечётное (синус) решения дифференциального уравнения
- [math]\displaystyle{ \frac{d^2}{d\varphi^2}R(\varphi) = - R(\varphi), }[/math]
с дополнительными условиями: [math]\displaystyle{ R(0) = 1 }[/math] для косинуса и [math]\displaystyle{ R'(0) = 1 }[/math] для синуса.
Определение как решений функциональных уравнений
Функции косинус и синус можно определить[5] как решения ([math]\displaystyle{ f }[/math] и [math]\displaystyle{ g }[/math] соответственно) системы функциональных уравнений:
[math]\displaystyle{ \left\{ \begin{array}{rcl} f(x+y)&=&f(x)f(y)-g(x)g(y)\\ g(x+y)&=&g(x)f(y)+f(x)g(y) \end{array} \right. }[/math]
при дополнительных условиях:
[math]\displaystyle{ f(x)^2 + g(x)^2 = 1, }[/math] [math]\displaystyle{ g(\pi/2) = 1, }[/math] и [math]\displaystyle{ 0 \lt g(x) \lt 1 }[/math] при [math]\displaystyle{ 0\lt x\lt \pi/2 }[/math].
Определение через ряды
Используя геометрию и свойства пределов, можно доказать, что производная синуса равна косинусу, и что производная косинуса равна минус синусу. Тогда можно воспользоваться теорией рядов Тейлора и представить синус и косинус в виде степенны́х рядов:
- [math]\displaystyle{ \sin x=x-\frac{x^3}{3!}+\frac{x^5}{5!}-\frac{x^7}{7!}+\frac{x^9}{9!}-\cdots = \sum_{n=0}^\infty\frac{(-1)^nx^{2n+1}}{(2n+1)!}, }[/math]
- [math]\displaystyle{ \cos x=1-\frac{x^2}{2!}+\frac{x^4}{4!}-\frac{x^6}{6!}+\frac{x^8}{8!}-\cdots = \sum_{n=0}^\infty\frac{(-1)^nx^{2n}}{(2n)!}. }[/math]
Пользуясь этими формулами, а также равенствами [math]\displaystyle{ \operatorname{tg}\,x=\frac{\sin x}{\cos x}, }[/math] [math]\displaystyle{ \operatorname{ctg}\,x=\frac{\cos x}{\sin x}, }[/math] [math]\displaystyle{ \sec x=\frac{1}{\cos x} }[/math] и [math]\displaystyle{ \operatorname{cosec}\,x=\frac{1}{\sin x}, }[/math] можно найти разложения в ряд и других тригонометрических функций:
- [math]\displaystyle{ {\operatorname{tg}\,x=x+\frac{1}{3}\,x^3 + \frac{2}{15}\,x^5 + \frac{17}{315}\,x^7 + \frac{62}{2835}\,x^9 + \cdots = \sum_{n=1}^\infty\frac{2^{2n}(2^{2n}-1)|B_{2n}|}{(2n)!}x^{2n-1} \quad \left(-\frac{\pi}{2}\lt x\lt \frac{\pi}{2}\right),} }[/math]
- [math]\displaystyle{ {\operatorname{ctg}\,x = \frac{1}{x} - \frac{x}{3} - \frac{x^3}{45} - \frac{2x^5}{945} - \frac{x^7}{4725} - \cdots = \frac{1}{x} - \sum_{n=1}^\infty \frac{2^{2n}|B_{2n}|}{(2n)!}\,x^{2n-1} \quad \left(-\pi \lt x \lt \pi\right),} }[/math]
- [math]\displaystyle{ {\sec x=1+\frac{1}{2}\,x^2+\frac{5}{24}\,x^4+\frac{61}{720}\,x^6+\frac{277}{8064}\,x^8+\cdots = \sum_{n=0}^\infty\frac{|E_{n}|}{(2n)!}\,x^{2n}, \quad \left(-\frac{\pi}{2} \lt x \lt \frac{\pi}{2}\right),} }[/math]
- [math]\displaystyle{ \operatorname{cosec} x = \frac{1}{x} + \frac{1}{6}\,x + \frac{7}{360}\,x^3 + \frac{31}{15120}\,x^5 + \frac{127}{604800}\,x^7 + \cdots = \frac{1}{x} + \sum_{n=1}^\infty \frac{2(2^{2n-1}-1) |B_{2n}|}{(2n)!}\,x^{2n-1} \quad \left(-\pi \lt x \lt \pi\right), }[/math]
где
- [math]\displaystyle{ B_n }[/math] — числа Бернулли,
- [math]\displaystyle{ E_n }[/math] — числа Эйлера.
Значения тригонометрических функций для некоторых углов
Значения синуса, косинуса, тангенса, котангенса, секанса и косеканса для некоторых углов приведены в таблице. («[math]\displaystyle{ \infty }[/math]» означает, что функция в указанной точке не определена, а в её окрестности стремится к бесконечности).

| Радианы | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ \frac{\pi}{6} }[/math] | [math]\displaystyle{ \frac{\pi}{4} }[/math] | [math]\displaystyle{ \frac{\pi}{3} }[/math] | [math]\displaystyle{ \frac{\pi}{2} }[/math] | [math]\displaystyle{ \pi }[/math] | [math]\displaystyle{ \frac{3\pi}{2} }[/math] | [math]\displaystyle{ 2\pi }[/math] |
|---|---|---|---|---|---|---|---|---|
| Градусы | [math]\displaystyle{ 0^\circ }[/math] | [math]\displaystyle{ 30^\circ }[/math] | [math]\displaystyle{ 45^\circ }[/math] | [math]\displaystyle{ 60^\circ }[/math] | [math]\displaystyle{ 90^\circ }[/math] | [math]\displaystyle{ 180^\circ }[/math] | [math]\displaystyle{ 270^\circ }[/math] | [math]\displaystyle{ 360^\circ }[/math] |
| [math]\displaystyle{ \sin \alpha }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ \frac{1}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{3}}{2} }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ 0 }[/math] |
| [math]\displaystyle{ \cos \alpha }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ \frac{\sqrt{3}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ \frac{1}{2} }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ 1 }[/math] |
| [math]\displaystyle{ \operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ \frac{1}{\sqrt{3}} }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ \sqrt{3} }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ 0 }[/math] |
| [math]\displaystyle{ \operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ \sqrt{3} }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ \frac{\sqrt{3}}{3} }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ 0 }[/math] | [math]\displaystyle{ \infty }[/math] |
| [math]\displaystyle{ \sec \alpha }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ \frac{2 \sqrt{3}}{3} }[/math] | [math]\displaystyle{ \sqrt{2} }[/math] | [math]\displaystyle{ 2 }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ 1 }[/math] |
| [math]\displaystyle{ \operatorname{cosec}\,\alpha }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ 2 }[/math] | [math]\displaystyle{ \sqrt{2} }[/math] | [math]\displaystyle{ \frac{2 \sqrt{3}}{3} }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ \infty }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ \infty }[/math] |
Значения тригонометрических функций нестандартных углов
| Радианы | [math]\displaystyle{ \frac{2\pi}{3} }[/math] | [math]\displaystyle{ \frac{3\pi}{4} }[/math] | [math]\displaystyle{ \frac{5\pi}{6} }[/math] | [math]\displaystyle{ \frac{7\pi}{6} }[/math] | [math]\displaystyle{ \frac{5\pi}{4} }[/math] | [math]\displaystyle{ \frac{4\pi}{3} }[/math] | [math]\displaystyle{ \frac{5\pi}{3} }[/math] | [math]\displaystyle{ \frac{7\pi}{4} }[/math] | [math]\displaystyle{ \frac{11\pi}{6} }[/math] |
|---|---|---|---|---|---|---|---|---|---|
| Градусы | [math]\displaystyle{ 120^\circ }[/math] | [math]\displaystyle{ 135^\circ }[/math] | [math]\displaystyle{ 150^\circ }[/math] | [math]\displaystyle{ 210^\circ }[/math] | [math]\displaystyle{ 225^\circ }[/math] | [math]\displaystyle{ 240^\circ }[/math] | [math]\displaystyle{ 300^\circ }[/math] | [math]\displaystyle{ 315^\circ }[/math] | [math]\displaystyle{ 330^\circ }[/math] |
| [math]\displaystyle{ \sin \alpha }[/math] | [math]\displaystyle{ \frac{\sqrt{3}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ \frac{1}{2} }[/math] | [math]\displaystyle{ -\frac{1}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ -\frac{1}{2} }[/math] |
| [math]\displaystyle{ \cos \alpha }[/math] | [math]\displaystyle{ -\frac{1}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{2} }[/math] | [math]\displaystyle{ -\frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ -\frac{1}{2} }[/math] | [math]\displaystyle{ \frac{1}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{2}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{3}}{2} }[/math] |
| [math]\displaystyle{ \operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ -\sqrt{3} }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{3} }[/math] | [math]\displaystyle{ \frac{\sqrt{3}}{3} }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ \sqrt{3} }[/math] | [math]\displaystyle{ -\sqrt{3} }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{3} }[/math] |
| [math]\displaystyle{ \operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{3} }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ -\sqrt{3} }[/math] | [math]\displaystyle{ \sqrt{3} }[/math] | [math]\displaystyle{ 1 }[/math] | [math]\displaystyle{ \frac{\sqrt{3}}{3} }[/math] | [math]\displaystyle{ -\frac{\sqrt{3}}{3} }[/math] | [math]\displaystyle{ -1 }[/math] | [math]\displaystyle{ -\sqrt{3} }[/math] |
| [math]\displaystyle{ \sec \alpha }[/math] | [math]\displaystyle{ -2 }[/math] | [math]\displaystyle{ -\sqrt{2} }[/math] | [math]\displaystyle{ -\frac{2 \sqrt{3}}{3} }[/math] | [math]\displaystyle{ -\frac{2 \sqrt{3}}{3} }[/math] | [math]\displaystyle{ -\sqrt{2} }[/math] | [math]\displaystyle{ -2 }[/math] | [math]\displaystyle{ 2 }[/math] | [math]\displaystyle{ \sqrt{2} }[/math] | [math]\displaystyle{ \frac{2 \sqrt{3}}{3} }[/math] |
| [math]\displaystyle{ \operatorname{cosec}\,\alpha }[/math] | [math]\displaystyle{ \frac{2 \sqrt{3}}{3} }[/math] | [math]\displaystyle{ \sqrt{2} }[/math] | [math]\displaystyle{ 2 }[/math] | [math]\displaystyle{ -2 }[/math] | [math]\displaystyle{ -\sqrt{2} }[/math] | [math]\displaystyle{ -\frac{2 \sqrt{3}}{3} }[/math] | [math]\displaystyle{ -\frac{2 \sqrt{3}}{3} }[/math] | [math]\displaystyle{ -\sqrt{2} }[/math] | [math]\displaystyle{ -2 }[/math] |
| Радианы | [math]\displaystyle{ \frac{\pi}{12} }[/math] | [math]\displaystyle{ \frac{\pi}{10} }[/math] | [math]\displaystyle{ \frac{\pi}{8} }[/math] | [math]\displaystyle{ \frac{\pi}{5} }[/math] | [math]\displaystyle{ \frac{3\pi}{10} }[/math] | [math]\displaystyle{ \frac{3\pi}{8} }[/math] | [math]\displaystyle{ \frac{2\pi}{5} }[/math] | [math]\displaystyle{ \frac{5\pi}{12} }[/math] |
|---|---|---|---|---|---|---|---|---|
| Градусы | [math]\displaystyle{ 15^\circ }[/math] | [math]\displaystyle{ 18^\circ }[/math] | [math]\displaystyle{ 22{,}5^\circ }[/math] | [math]\displaystyle{ 36^\circ }[/math] | [math]\displaystyle{ 54^\circ }[/math] | [math]\displaystyle{ 67{,}5^\circ }[/math] | [math]\displaystyle{ 72^\circ }[/math] | [math]\displaystyle{ 75^\circ }[/math] |
| [math]\displaystyle{ \sin \alpha }[/math] | [math]\displaystyle{ \frac{\sqrt{2-\sqrt{3}}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{5}-1}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{2-\sqrt{2}}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{10-2\sqrt{5}}}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{5}+1}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{2+\sqrt{2}}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{10+2\sqrt{5}}}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{2+\sqrt{3}}}{2} }[/math] |
| [math]\displaystyle{ \cos \alpha }[/math] | [math]\displaystyle{ \frac{\sqrt{2+\sqrt{3}}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{10+2\sqrt{5}}}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{2+\sqrt{2}}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{5}+1}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{10-2\sqrt{5}}}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{2-\sqrt{2}}}{2} }[/math] | [math]\displaystyle{ \frac{\sqrt{5}-1}{4} }[/math] | [math]\displaystyle{ \frac{\sqrt{2-\sqrt{3}}}{2} }[/math] |
| [math]\displaystyle{ \operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ 2-\sqrt{3} }[/math] | [math]\displaystyle{ \frac{\sqrt{25-10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ \sqrt{2}-1 }[/math] | [math]\displaystyle{ \sqrt{5-2\sqrt{5}} }[/math] | [math]\displaystyle{ \frac{\sqrt{25+10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ \sqrt{2}+1 }[/math] | [math]\displaystyle{ \sqrt{5+2\sqrt{5}} }[/math] | [math]\displaystyle{ 2+\sqrt{3} }[/math] |
| [math]\displaystyle{ \operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ 2+\sqrt{3} }[/math] | [math]\displaystyle{ \sqrt{5+2\sqrt{5}} }[/math] | [math]\displaystyle{ \sqrt{2}+1 }[/math] | [math]\displaystyle{ \frac{\sqrt{25+10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ \sqrt{5-2\sqrt{5}} }[/math] | [math]\displaystyle{ \sqrt{2}-1 }[/math] | [math]\displaystyle{ \frac{\sqrt{25-10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ 2-\sqrt{3} }[/math] |
| [math]\displaystyle{ \sec \alpha }[/math] | [math]\displaystyle{ 2\sqrt{2-\sqrt{3}} }[/math] | [math]\displaystyle{ \frac{\sqrt{50-10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ \sqrt{4-2\sqrt{2}} }[/math] | [math]\displaystyle{ \sqrt{5}-1 }[/math] | [math]\displaystyle{ \frac{\sqrt{50+10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ \sqrt{4+2\sqrt{2}} }[/math] | [math]\displaystyle{ \sqrt{5}+1 }[/math] | [math]\displaystyle{ 2\sqrt{2+\sqrt{3}} }[/math] |
| [math]\displaystyle{ \operatorname{cosec}\,\alpha }[/math] | [math]\displaystyle{ 2\sqrt{2+\sqrt{3}} }[/math] | [math]\displaystyle{ \sqrt{5}+1 }[/math] | [math]\displaystyle{ \sqrt{4+2\sqrt{2}} }[/math] | [math]\displaystyle{ \frac{\sqrt{50+10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ \sqrt{5}-1 }[/math] | [math]\displaystyle{ \sqrt{4-2\sqrt{2}} }[/math] | [math]\displaystyle{ \frac{\sqrt{50-10\sqrt{5}}}{5} }[/math] | [math]\displaystyle{ 2\sqrt{2-\sqrt{3}} }[/math] |
[math]\displaystyle{ \sin \frac{\pi}{60} = \cos \frac{29\,\pi}{60} = \sin 3^\circ = \cos 87^\circ = \frac{\sqrt{2}(\sqrt{3}+1)(\sqrt{5}-1)-2(\sqrt{3}-1)\sqrt{5+\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \cos \frac{\pi}{60} = \sin \frac{29\,\pi}{60} = \cos 3^\circ = \sin 87^\circ = \frac{\sqrt{2}(\sqrt{3}-1)(\sqrt{5}-1)+2(\sqrt{3}+1)\sqrt{5+\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{\pi}{60} = \operatorname{ctg} \frac{29\,\pi}{60} = \operatorname{tg} 3^\circ = \operatorname{ctg} 87^\circ = \frac{2(\sqrt{5}+2)-\sqrt{3}(\sqrt{5}+3)+(2-\sqrt{3})(\sqrt{3}(\sqrt{5}+1)-2)\sqrt{5-2\sqrt{5}}}{2}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{\pi}{60} = \operatorname{tg} \frac{29\,\pi}{60} = \operatorname{ctg} 3^\circ = \operatorname{tg} 87^\circ = \frac{2(2(\sqrt{5}+2)+\sqrt{3}(\sqrt{5}+3))+(\sqrt{3}(\sqrt{5}-1)+2)\sqrt{2(25+11\sqrt{5})}}{4}, }[/math]
[math]\displaystyle{ \sin \frac{\pi}{30} = \cos \frac{7\,\pi}{15} = \sin 6^\circ = \cos 84^\circ = \frac{\sqrt{6(5-\sqrt{5})}-\sqrt{5}-1}{8}, }[/math]
[math]\displaystyle{ \cos \frac{\pi}{30} = \sin \frac{7\,\pi}{15} = \cos 6^\circ = \sin 84^\circ = \frac{\sqrt{2(5-\sqrt{5})}+\sqrt{3}(\sqrt{5}+1)}{8}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{\pi}{30} = \operatorname{ctg} \frac{7\,\pi}{15} = \operatorname{tg} 6^\circ = \operatorname{ctg} 84^\circ = \frac{\sqrt{2(5-\sqrt{5})}-\sqrt{3}(\sqrt{5}-1)}{2}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{\pi}{30} = \operatorname{tg} \frac{7\,\pi}{15} = \operatorname{ctg} 6^\circ = \operatorname{tg} 84^\circ = \frac{\sqrt{2(25+11\sqrt{5})}+\sqrt{3}(\sqrt{5}+3)}{2}, }[/math]
[math]\displaystyle{ \sin \frac{\pi}{20} = \cos \frac{9\,\pi}{20} = \sin 9^\circ = \cos 81^\circ = \frac{\sqrt{2}(\sqrt{5}+1)-2\sqrt{5-\sqrt{5}}}{8}, }[/math]
[math]\displaystyle{ \cos \frac{\pi}{20} = \sin \frac{9\,\pi}{20} = \cos 9^\circ = \sin 81^\circ = \frac{\sqrt{2}(\sqrt{5}+1)+2\sqrt{5-\sqrt{5}}}{8}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{\pi}{20} = \operatorname{ctg} \frac{9\,\pi}{20} = \operatorname{tg} 9^\circ = \operatorname{ctg} 81^\circ = {\sqrt{5}+1-\sqrt{5+2\sqrt{5}}}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{\pi}{20} = \operatorname{tg} \frac{9\,\pi}{20} = \operatorname{ctg} 9^\circ = \operatorname{tg} 81^\circ = {\sqrt{5}+1+\sqrt{5+2\sqrt{5}}}, }[/math]
[math]\displaystyle{ \sin \frac{\pi}{15} = \cos \frac{13\,\pi}{30} = \sin 12^\circ = \cos 78^\circ = \frac{\sqrt{2(5+\sqrt{5})}-\sqrt{3}(\sqrt{5}-1)}{8}, }[/math]
[math]\displaystyle{ \cos \frac{\pi}{15} = \sin \frac{13\,\pi}{30} = \cos 12^\circ = \sin 78^\circ = \frac{\sqrt{6(5+\sqrt{5})}+\sqrt{5}-1}{8}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{\pi}{15} = \operatorname{ctg} \frac{13\,\pi}{30} = \operatorname{tg} 12^\circ = \operatorname{ctg} 78^\circ = \frac{\sqrt{3}(3-\sqrt{5})-\sqrt{2(25-11\sqrt{5})}}{2}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{\pi}{15} = \operatorname{tg} \frac{13\,\pi}{30} = \operatorname{ctg} 12^\circ = \operatorname{tg} 78^\circ = \frac{\sqrt{3}(\sqrt{5}+1)+\sqrt{2(5+\sqrt{5})}}{2}, }[/math]
[math]\displaystyle{ \sin \frac{7\,\pi}{60} = \cos \frac{23\,\pi}{60} = \sin 21^\circ = \cos 69^\circ = \frac{-\sqrt{2}(\sqrt{3}-1)(\sqrt{5}+1)+2(\sqrt{3}+1)\sqrt{5-\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \cos \frac{7\,\pi}{60} = \sin \frac{23\,\pi}{60} = \cos 21^\circ = \sin 69^\circ = \frac{\sqrt{2}(\sqrt{3}+1)(\sqrt{5}+1)+2(\sqrt{3}-1)\sqrt{5-\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{7\,\pi}{60} = \operatorname{ctg} \frac{23\,\pi}{60} = \operatorname{tg} 21^\circ = \operatorname{ctg} 69^\circ = \frac{2(2(\sqrt{5}-2)-\sqrt{3}(3-\sqrt{5}))+(\sqrt{3}(\sqrt{5}+1)-2)\sqrt{2(25-11\sqrt{5})}}{4}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{7\,\pi}{60} = \operatorname{tg} \frac{23\,\pi}{60} = \operatorname{ctg} 21^\circ = \operatorname{tg} 69^\circ = \frac{2(2(\sqrt{5}-2)+\sqrt{3}(3-\sqrt{5}))+(\sqrt{3}(\sqrt{5}+1)+2)\sqrt{2(25-11\sqrt{5})}}{4}, }[/math]
[math]\displaystyle{ \sin \frac{2\,\pi}{15} = \cos \frac{11\,\pi}{30} = \sin 24^\circ = \cos 66^\circ = \frac{\sqrt{3}(\sqrt{5}+1)-\sqrt{2(5-\sqrt{5})}}{8}, }[/math]
[math]\displaystyle{ \cos \frac{2\,\pi}{15} = \sin \frac{11\,\pi}{30} = \cos 24^\circ = \sin 66^\circ = \frac{\sqrt{5}+1+\sqrt{6(5-\sqrt{5})}}{8}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{2\,\pi}{15} = \operatorname{ctg} \frac{11\,\pi}{30} = \operatorname{tg} 24^\circ = \operatorname{ctg} 66^\circ = \frac{-\sqrt{3}(3+\sqrt{5})+\sqrt{2(25+11\sqrt{5})}}{2}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{2\,\pi}{15} = \operatorname{tg} \frac{11\,\pi}{30} = \operatorname{ctg} 24^\circ = \operatorname{tg} 66^\circ = \frac{\sqrt{3}(\sqrt{5}-1)+\sqrt{2(5-\sqrt{5})}}{2}, }[/math]
[math]\displaystyle{ \sin \frac{3\,\pi}{20} = \cos \frac{7\,\pi}{20} = \sin 27^\circ = \cos 63^\circ = \frac{-\sqrt{2}(\sqrt{5}-1)+2\sqrt{5+\sqrt{5}}}{8}, }[/math]
[math]\displaystyle{ \cos \frac{3\,\pi}{20} = \sin \frac{7\,\pi}{20} = \cos 27^\circ = \sin 63^\circ = \frac{\sqrt{2}(\sqrt{5}-1)+2\sqrt{5+\sqrt{5}}}{8}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{3\,\pi}{20} = \operatorname{ctg} \frac{7\,\pi}{20} = \operatorname{tg} 27^\circ = \operatorname{ctg} 63^\circ = {\sqrt{5}-1-\sqrt{5-2\sqrt{5}}}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{3\,\pi}{20} = \operatorname{tg} \frac{7\,\pi}{20} = \operatorname{ctg} 27^\circ = \operatorname{tg} 63^\circ = {\sqrt{5}-1+\sqrt{5-2\sqrt{5}}}, }[/math]
[math]\displaystyle{ \sin \frac{11\,\pi}{60} = \cos \frac{19\,\pi}{60} = \sin 33^\circ = \cos 57^\circ = \frac{\sqrt{2}(\sqrt{3}+1)(\sqrt{5}-1)+2(\sqrt{3}-1)\sqrt{5+\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \cos \frac{11\,\pi}{60} = \sin \frac{19\,\pi}{60} = \cos 33^\circ = \sin 57^\circ = \frac{-\sqrt{2}(\sqrt{3}-1)(\sqrt{5}-1)+2(\sqrt{3}+1)\sqrt{5+\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{11\,\pi}{60} = \operatorname{ctg} \frac{19\,\pi}{60} = \operatorname{tg} 33^\circ = \operatorname{ctg} 57^\circ = \frac{-2(\sqrt{5}+2)+\sqrt{3}(3+\sqrt{5})+(2-\sqrt{3})(\sqrt{3}(\sqrt{5}+1)-2)\sqrt{5-2\sqrt{5}}}{2}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{11\,\pi}{60} = \operatorname{tg} \frac{19\,\pi}{60} = \operatorname{ctg} 33^\circ = \operatorname{tg} 57^\circ = \frac{-2(2(\sqrt{5}+2)+\sqrt{3}(3+\sqrt{5}))+(\sqrt{3}(\sqrt{5}-1)+2)\sqrt{2(25+11\sqrt{5})}}{4}, }[/math]
[math]\displaystyle{ \sin \frac{13\,\pi}{60} = \cos \frac{17\,\pi}{60} = \sin 39^\circ = \cos 51^\circ = \frac{\sqrt{2}(\sqrt{3}+1)(\sqrt{5}+1)-2(\sqrt{3}-1)\sqrt{5-\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \cos \frac{13\,\pi}{60} = \sin \frac{17\,\pi}{60} = \cos 39^\circ = \sin 51^\circ = \frac{\sqrt{2}(\sqrt{3}-1)(\sqrt{5}+1)+2(\sqrt{3}+1)\sqrt{5-\sqrt{5}}}{16}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{13\,\pi}{60} = \operatorname{ctg} \frac{17\,\pi}{60} = \operatorname{tg} 39^\circ = \operatorname{ctg} 51^\circ = \frac{-2(2(\sqrt{5}-2)+\sqrt{3}(3-\sqrt{5}))+(\sqrt{3}(\sqrt{5}+1)+2)\sqrt{2(25-11\sqrt{5})}}{4}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{13\,\pi}{60} = \operatorname{tg} \frac{17\,\pi}{60} = \operatorname{ctg} 39^\circ = \operatorname{tg} 51^\circ = \frac{-2(2(\sqrt{5}-2)-\sqrt{3}(3-\sqrt{5}))+(\sqrt{3}(\sqrt{5}+1)-2)\sqrt{2(25-11\sqrt{5})}}{4}, }[/math]
[math]\displaystyle{ \sin \frac{7\,\pi}{30} = \cos \frac{8\,\pi}{30} = \sin 42^\circ = \cos 48^\circ = \frac{-(\sqrt{5}-1)+\sqrt{6(5+\sqrt{5})}}{8}, }[/math]
[math]\displaystyle{ \cos \frac{7\,\pi}{30} = \sin \frac{8\,\pi}{30} = \cos 42^\circ = \sin 48^\circ = \frac{\sqrt{3}(\sqrt{5}-1)+\sqrt{2(5+\sqrt{5})}}{8}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{7\,\pi}{30} = \operatorname{ctg} \frac{8\,\pi}{30} = \operatorname{tg} 42^\circ = \operatorname{ctg} 48^\circ = \frac{\sqrt{3}(\sqrt{5}+1)-\sqrt{2(5+\sqrt{5})}}{2}, }[/math]
[math]\displaystyle{ \operatorname{ctg} \frac{7\,\pi}{30} = \operatorname{tg} \frac{8\,\pi}{30} = \operatorname{ctg} 42^\circ = \operatorname{tg} 48^\circ = \frac{\sqrt{3}(3-\sqrt{5})+\sqrt{2(25-11\sqrt{5})}}{2}, }[/math]
[math]\displaystyle{ \operatorname{tg} \frac{\pi}{120} = \operatorname{ctg} \frac{59\,\pi}{120} = \operatorname{tg} 1.5^\circ = \operatorname{ctg} 88.5^\circ = \sqrt{\frac{8-\sqrt{2(2-\sqrt{3})(3-\sqrt{5})} - \sqrt{ 2(2+\sqrt{3})(5+\sqrt{5})}}{8+\sqrt{2(2-\sqrt{3})(3-\sqrt{5})}+\sqrt{2(2+\sqrt{3})(5+\sqrt{5})} }}, }[/math]
[math]\displaystyle{ \cos \frac{\pi}{240} = \sin \frac{119\,\pi}{240} = \cos 0.75^\circ = \sin 89.25^\circ = \frac{1}{16} \left( \sqrt{2-\sqrt{2+\sqrt{2}}} \left(\sqrt{2(5+\sqrt{5})}+\sqrt{3}(1-\sqrt{5}) \right) + \sqrt{2+\sqrt{2+\sqrt{2}}} \left (\sqrt{6(5+\sqrt{5})}+\sqrt{5} - 1 \right) \right), }[/math]
[math]\displaystyle{ \cos \frac{\pi}{17} = \sin \frac{15\,\pi}{34} = \frac{1}{8}\sqrt{2 \left(2\sqrt{3\sqrt{17}-\sqrt{2(85+19\sqrt{17})} +17}+\sqrt{2(17-\sqrt{17})}+\sqrt{17}+15 \right)}. }[/math]
[math]\displaystyle{ \sin{\pi\over2^{n+1}}={1\over2}\underbrace{\sqrt{2-\sqrt{2+\dots+\sqrt{2}}}}_{n}, n\in\mathbb N }[/math]
[math]\displaystyle{ \cos{\pi\over2^{n+1}}={1\over2}\underbrace{\sqrt{2+\sqrt{2+\dots+\sqrt{2}}}}_{n}, n\in\mathbb N }[/math]
[math]\displaystyle{ \sin{\pi\over3\cdot2^{n}}={1\over2}\underbrace{\sqrt{2-\sqrt{2+\dots+\sqrt{3}}}}_{n}, n\geq 2 }[/math]
[math]\displaystyle{ \cos{\pi\over3\cdot2^{n}}={1\over2}\underbrace{\sqrt{2+\sqrt{2+\dots+\sqrt{3}}}}_{n}, n\geq 2 }[/math]
Свойства тригонометрических функций
Простейшие тождества
Поскольку синус и косинус являются соответственно ординатой и абсциссой точки, соответствующей на единичной окружности углу α, то, согласно уравнению единичной окружности ([math]\displaystyle{ x^2+y^2=1 }[/math]) или теореме Пифагора, имеем:
- [math]\displaystyle{ \sin^2 \alpha + \cos^2 \alpha = 1. }[/math]
Это соотношение называется основным тригонометрическим тождеством.
Разделив это уравнение на квадрат косинуса и синуса соответственно, получим:
- [math]\displaystyle{ 1 + \mathop{\mathrm{tg}}\,^2 \alpha = \mathop{\mathrm{sec}}\,^2 \alpha, }[/math]
- [math]\displaystyle{ 1 + \mathop{\mathrm{ctg}}\,^2 \alpha = \mathop{\mathrm{cosec}}\,^2 \alpha. }[/math]
Из определения тангенса и котангенса следует, что
- [math]\displaystyle{ \mathop{\mathrm{tg}}\,\alpha \cdot \mathop{\mathrm{ctg}}\,\alpha=1. }[/math]
Любую тригонометрическую функцию можно выразить через любую другую тригонометрическую функцию с тем же аргументом (с точностью до знака из-за неоднозначности раскрытия квадратного корня). Нижеприведённые формулы верны для [math]\displaystyle{ 0\lt x\lt \pi/2 }[/math]:
| sin | cos | tg | ctg | sec | cosec | |
|---|---|---|---|---|---|---|
| [math]\displaystyle{ \,\sin x = }[/math] | [math]\displaystyle{ \,\sin x }[/math] | [math]\displaystyle{ \sqrt{1-\cos^2 x } }[/math] | [math]\displaystyle{ \frac{\operatorname{tg} x }{\sqrt{1 + \operatorname{tg}^2 x }} }[/math] | [math]\displaystyle{ \frac{1}{\sqrt{\operatorname{ctg}^2 x + 1}} }[/math] | [math]\displaystyle{ \frac{\sqrt{\sec^2 x -1}} {\sec x } }[/math] | [math]\displaystyle{ \frac{1}{\operatorname{cosec} x } }[/math] |
| [math]\displaystyle{ \, \cos x = }[/math] | [math]\displaystyle{ \, \sqrt{1-\sin^2 x } }[/math] | [math]\displaystyle{ \, \cos x }[/math] | [math]\displaystyle{ \, \frac{1}{\sqrt{1 + \operatorname{tg}^2 x }} }[/math] | [math]\displaystyle{ \, \frac{\operatorname{ctg} x } {\sqrt{\operatorname{ctg}^2 x + 1}} }[/math] | [math]\displaystyle{ \, \frac{1}{\sec x } }[/math] | [math]\displaystyle{ \, \frac{\sqrt{\operatorname{cosec}^2 x -1}}{\operatorname{cosec} x } }[/math] |
| [math]\displaystyle{ \, \operatorname{tg} x = }[/math] | [math]\displaystyle{ \, \frac{\sin x }{\sqrt{1-\sin^2 x }} }[/math] | [math]\displaystyle{ \, \frac{\sqrt{1-\cos^2 x }}{\cos x } }[/math] | [math]\displaystyle{ \, \operatorname{tg} x }[/math] | [math]\displaystyle{ \, \frac{1}{\operatorname{ctg} x } }[/math] | [math]\displaystyle{ \, \sqrt{\sec^2 x - 1} }[/math] | [math]\displaystyle{ \, \frac{1}{ \sqrt{\operatorname{cosec}^2 x -1}} }[/math] |
| [math]\displaystyle{ \, \operatorname{ctg}x = }[/math] | [math]\displaystyle{ \, \frac{\sqrt{1-\sin^2 x }}{\sin x } }[/math] | [math]\displaystyle{ \, \frac{\cos x }{\sqrt{1-\cos^2 x }} }[/math] | [math]\displaystyle{ \, \frac{1}{\operatorname{tg} x } }[/math] | [math]\displaystyle{ \, \operatorname{ctg}x }[/math] | [math]\displaystyle{ \, \frac{1}{\sqrt{\sec^2 x - 1}} }[/math] | [math]\displaystyle{ \, \sqrt{\operatorname{cosec}^2 x - 1} }[/math] |
| [math]\displaystyle{ \, \sec x = }[/math] | [math]\displaystyle{ \, \frac{1}{\sqrt{1-\sin^2 x }} }[/math] | [math]\displaystyle{ \, \frac{1}{\cos x } }[/math] | [math]\displaystyle{ \, \sqrt{1 + \operatorname{tg}^2 x } }[/math] | [math]\displaystyle{ \, \frac{\sqrt{\operatorname{ctg}^2 x + 1}}{\operatorname{ctg} x } }[/math] | [math]\displaystyle{ \, \sec x }[/math] | [math]\displaystyle{ \, \frac{\operatorname{cosec} x }{\sqrt{\operatorname{cosec}^2 x - 1}} }[/math] |
| [math]\displaystyle{ \, \operatorname{cosec} x = }[/math] | [math]\displaystyle{ \, \frac{1}{\sin x } }[/math] | [math]\displaystyle{ \, \frac{1}{\sqrt{1 - \cos^2 x }} }[/math] | [math]\displaystyle{ \, \frac{\sqrt{1 + \operatorname{tg}^2 x }} {\operatorname{tg} x } }[/math] | [math]\displaystyle{ \, \sqrt{\operatorname{ctg}^2 x + 1} }[/math] | [math]\displaystyle{ \, \frac{\sec x }{\sqrt{\sec^2 x - 1}} }[/math] | [math]\displaystyle{ \, \operatorname{cosec} x }[/math] |
Непрерывность
- Синус и косинус — непрерывные функции.
- Тангенс и секанс имеют точки разрыва [math]\displaystyle{ \pi/2+\pi k }[/math], где [math]\displaystyle{ k }[/math] — любое целое.
- Котангенс и косеканс имеют точки разрыва [math]\displaystyle{ \pi k }[/math], где [math]\displaystyle{ k }[/math] — любое целое.
Чётность
Косинус и секанс — чётные. Остальные четыре функции — нечётные, то есть:
- [math]\displaystyle{ \sin \left( - \alpha \right) = - \sin \alpha \,, }[/math]
- [math]\displaystyle{ \cos \left( - \alpha \right) = \cos \alpha \,, }[/math]
- [math]\displaystyle{ \mathop{\mathrm{tg}}\, \left( - \alpha \right) = - \mathop{\mathrm{tg}}\, \alpha \,, }[/math]
- [math]\displaystyle{ \mathop{\mathrm{ctg}}\, \left( - \alpha \right) = - \mathop{\mathrm{ctg}}\, \alpha \,, }[/math]
- [math]\displaystyle{ \sec \left( - \alpha \right) = \sec \alpha \,, }[/math]
- [math]\displaystyle{ \mathop{\mathrm{cosec}}\, \left( - \alpha \right) = - \mathop{\mathrm{cosec}}\, \alpha \,. }[/math]
Периодичность
Функции [math]\displaystyle{ \sin x,\; \cos x,\; \sec x,\; \mathrm{cosec}\, x }[/math] — периодические с периодом [math]\displaystyle{ 2\pi }[/math], функции [math]\displaystyle{ \mathrm{tg}\, x }[/math] и [math]\displaystyle{ \mathrm{ctg}\, x }[/math] — c периодом [math]\displaystyle{ \pi }[/math].
Формулы приведения
Формулами приведения называются формулы следующего вида:
- [math]\displaystyle{ f ( n \pi + \alpha ) = \pm f (\alpha), }[/math]
- [math]\displaystyle{ f ( n \pi - \alpha ) = \pm f (\alpha), }[/math]
- [math]\displaystyle{ f \left( \frac{(2n+1) \pi}{2} + \alpha\right) = \pm g (\alpha), }[/math]
- [math]\displaystyle{ f \left( \frac{(2n+1) \pi}{2} - \alpha\right) = \pm g (\alpha). }[/math]
Здесь [math]\displaystyle{ f }[/math] — любая тригонометрическая функция, [math]\displaystyle{ g }[/math] — соответствующая ей кофункция (то есть косинус для синуса, синус для косинуса, тангенс для котангенса, котангенс для тангенса, секанс для косеканса и косеканс для секанса), [math]\displaystyle{ n }[/math] — целое число. Перед полученной функцией ставится тот знак, который имеет исходная функция в заданной координатной четверти при условии, что угол [math]\displaystyle{ \alpha }[/math] острый, например:
- [math]\displaystyle{ \cos \left( \frac{ \pi}{2} - \alpha \right) = \sin \alpha\,, }[/math] или что то же самое: [math]\displaystyle{ \cos \left( 90^\circ - \alpha \right) = \sin \alpha\,. }[/math]
Некоторые формулы приведения:
| [math]\displaystyle{ \alpha }[/math] | [math]\displaystyle{ \frac{\pi}{2} - \alpha }[/math] | [math]\displaystyle{ \frac{\pi}{2} + \alpha }[/math] | [math]\displaystyle{ \pi - \alpha }[/math] | [math]\displaystyle{ \pi + \alpha }[/math] | [math]\displaystyle{ \frac{3\,\pi}{2} - \alpha }[/math] | [math]\displaystyle{ \frac{3\,\pi}{2} + \alpha }[/math] | [math]\displaystyle{ 2\,\pi - \alpha }[/math] |
| [math]\displaystyle{ \sin\alpha }[/math] | [math]\displaystyle{ \cos\alpha }[/math] | [math]\displaystyle{ \cos\alpha }[/math] | [math]\displaystyle{ \sin\alpha }[/math] | [math]\displaystyle{ -\sin\alpha }[/math] | [math]\displaystyle{ -\cos\alpha }[/math] | [math]\displaystyle{ -\cos\alpha }[/math] | [math]\displaystyle{ -\sin\alpha }[/math] |
| [math]\displaystyle{ \cos\alpha }[/math] | [math]\displaystyle{ \sin\alpha }[/math] | [math]\displaystyle{ -\sin\alpha }[/math] | [math]\displaystyle{ -\cos\alpha }[/math] | [math]\displaystyle{ -\cos\alpha }[/math] | [math]\displaystyle{ -\sin\alpha }[/math] | [math]\displaystyle{ \sin\alpha }[/math] | [math]\displaystyle{ \cos\alpha }[/math] |
| [math]\displaystyle{ \operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ \operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ \operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ \operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{tg}\,\alpha }[/math] |
| [math]\displaystyle{ \operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ \operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ \operatorname{ctg}\,\alpha }[/math] | [math]\displaystyle{ \operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{tg}\,\alpha }[/math] | [math]\displaystyle{ -\operatorname{ctg}\,\alpha }[/math] |
Интересующие формулы приведения так же могут легко быть получены рассмотрением функций на единичной окружности.
Формулы сложения и вычитания
Значения тригонометрических функций суммы и разности двух углов:
- [math]\displaystyle{ \sin\left( \alpha \pm \beta \right)= \sin\alpha \, \cos\beta \pm \cos\alpha \, \sin\beta, }[/math]
- [math]\displaystyle{ \cos\left( \alpha \pm \beta \right)= \cos\alpha \, \cos\beta \mp \sin\alpha \, \sin\beta, }[/math]
- [math]\displaystyle{ \operatorname{tg}\left( \alpha \pm \beta \right) = \frac{\operatorname{tg}\,\alpha \pm \operatorname{tg}\,\beta}{1 \mp \operatorname{tg}\,\alpha \, \operatorname{tg}\,\beta}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\left( \alpha \pm \beta \right) = \frac{\operatorname{ctg}\,\alpha\,\operatorname{ctg}\,\beta \mp 1}{\operatorname{ctg}\,\beta \pm \operatorname{ctg}\,\alpha}. }[/math]
Аналогичные формулы для суммы трёх углов:
- [math]\displaystyle{ \sin \left( \alpha + \beta + \gamma \right) = \sin \alpha \cos \beta \cos \gamma + \cos \alpha \sin \beta \cos \gamma + \cos \alpha \cos \beta \sin \gamma - \sin \alpha \sin \beta \sin \gamma, }[/math]
- [math]\displaystyle{ \cos \left( \alpha + \beta + \gamma \right) = \cos \alpha \cos \beta \cos \gamma - \sin \alpha \sin \beta \cos \gamma - \sin \alpha \cos \beta \sin \gamma - \cos \alpha \sin \beta \sin \gamma. }[/math]
Формулы для кратных углов
Формулы двойного угла:
- [math]\displaystyle{ \sin 2\alpha = 2 \sin \alpha \cos \alpha = \frac{2\,\operatorname{tg}\,\alpha }{1 + \operatorname{tg}^2\alpha} = \frac{2\,\operatorname{ctg}\,\alpha }{1 + \operatorname{ctg}^2\alpha} = \frac{2}{\operatorname{tg}\,\alpha + \operatorname{ctg}\,\alpha}, }[/math]
- [math]\displaystyle{ \cos 2\alpha = \cos^2 \alpha\,-\,\sin^2 \alpha = 2 \cos^2 \alpha\,-\,1 = 1\,-\,2 \sin^2 \alpha = \frac{1 - \operatorname{tg}^2 \alpha}{1 + \operatorname{tg}^2\alpha} = \frac{\operatorname{ctg}^2 \alpha - 1}{\operatorname{ctg}^2\alpha + 1} = \frac{\operatorname{ctg}\,\alpha - \operatorname{tg}\,\alpha}{\operatorname{ctg}\,\alpha + \operatorname{tg}\,\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,2 \alpha = \frac{2\,\operatorname{tg}\,\alpha}{1 - \operatorname{tg}^2\alpha} = \frac{2\,\operatorname{ctg}\,\alpha}{\operatorname{ctg}^2\alpha - 1} = \frac{2}{\operatorname{ctg}\,\alpha - \operatorname{tg}\,\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\,2 \alpha = \frac{\operatorname{ctg}^2 \alpha - 1}{2\,\operatorname{ctg}\,\alpha} = \frac{\operatorname{ctg}\,\alpha - \operatorname{tg}\,\alpha}{2}. }[/math]
Формулы тройного угла:
- [math]\displaystyle{ \sin\,3\alpha=3\sin\alpha - 4\sin^3\alpha, }[/math]
- [math]\displaystyle{ \cos\,3\alpha=4\cos^3\alpha -3\cos\alpha, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,3\alpha=\frac{3\,\operatorname{tg}\,\alpha - \operatorname{tg}^3\,\alpha}{1 - 3\,\operatorname{tg}^2\,\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\,3\alpha=\frac{\operatorname{ctg}^3\,\alpha - 3\,\operatorname{ctg}\,\alpha}{3\,\operatorname{ctg}^2\,\alpha - 1}. }[/math]
Прочие формулы для кратных углов:
- [math]\displaystyle{ \sin\,4\alpha=\cos\alpha \left(4\sin\alpha - 8\sin^3\alpha\right), }[/math]
- [math]\displaystyle{ \cos\,4\alpha=8\cos^4\alpha - 8\cos^2\alpha + 1, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,4\alpha=\frac{4\,\operatorname{tg}\,\alpha - 4\,\operatorname{tg}^3\,\alpha}{1 - 6\,\operatorname{tg}^2\,\alpha + \operatorname{tg}^4\,\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\,4\alpha=\frac{\operatorname{ctg}^4\,\alpha - 6\,\operatorname{ctg}^2\,\alpha + 1}{4\,\operatorname{ctg}^3\,\alpha - 4\,\operatorname{ctg}\,\alpha}, }[/math]
- [math]\displaystyle{ \sin\,5\alpha=16\sin^5\alpha-20\sin^3\alpha +5\sin\alpha, }[/math]
- [math]\displaystyle{ \cos\,5\alpha=16\cos^5\alpha-20\cos^3\alpha +5\cos\alpha, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,5\alpha=\operatorname{tg}\alpha\frac{\operatorname{tg}^4\alpha-10\operatorname{tg}^2\alpha+5}{5\operatorname{tg}^4\alpha-10\operatorname{tg}^2\alpha+1}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\,5\alpha=\operatorname{ctg}\alpha\frac{\operatorname{ctg}^4\alpha-10\operatorname{ctg}^2\alpha+5}{5\operatorname{ctg}^4\alpha-10\operatorname{ctg}^2\alpha+1}, }[/math]
- [math]\displaystyle{ \sin (n\alpha)=2^{n-1}\prod^{n-1}_{k=0}\sin\left( \alpha+\frac{\pi k}{n}\right) }[/math] следует из формулы дополнения и формулы Гаусса для гамма-функции.
Из формулы Муавра можно получить следующие общие выражения для кратных углов:
- [math]\displaystyle{ \sin(n\alpha)=\sum_{k=0}^{[(n-1)/2]}(-1)^k\binom{n}{2k+1}\cos^{n-2k-1}\alpha\,\sin^{2k+1}\alpha, }[/math]
- [math]\displaystyle{ \cos(n\alpha)=\sum_{k=0}^{[n/2]}(-1)^k\binom{n}{2k}\cos^{n-2k}\alpha\,\sin^{2k}\alpha, }[/math]
- [math]\displaystyle{ \mathrm{tg}(n\alpha)=\frac{\sin(n\alpha)}{\cos(n\alpha)}=\dfrac{\displaystyle{\sum\limits_{k=0}^{[(n-1)/2]}(-1)^k\binom{n}{2k+1}\mathrm{tg}^{2k+1}\alpha}}{\displaystyle{\sum\limits_{k=0}^{[n/2]}(-1)^k\binom{n}{2k}\mathrm{tg}^{2k}\alpha}}, }[/math]
- [math]\displaystyle{ \mathrm{ctg}(n\alpha)=\frac{\cos(n\alpha)}{\sin(n\alpha)}=\dfrac{\displaystyle{\sum\limits_{k=0}^{[n/2]}(-1)^k\binom{n}{2k}\mathrm{ctg}^{n-2k}\alpha}}{\displaystyle{\sum\limits_{k=0}^{[(n-1)/2]}(-1)^k\binom{n}{2k+1}\mathrm{ctg}^{n-2k-1}\alpha}}, }[/math]
где [math]\displaystyle{ [n] }[/math] — целая часть числа [math]\displaystyle{ n }[/math], [math]\displaystyle{ \binom{n}{k} }[/math] — биномиальный коэффициент.
Формулы половинного угла:
- [math]\displaystyle{ \sin\frac{\alpha}{2}=\sqrt{\frac{1-\cos\alpha}{2}},\quad 0 \leqslant \alpha \leqslant 2\pi, }[/math]
- [math]\displaystyle{ \cos\frac{\alpha}{2}=\sqrt{\frac{1+\cos\alpha}{2}},\quad -\pi \leqslant \alpha \leqslant \pi, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,\frac{\alpha}{2}=\frac{1-\cos\alpha}{\sin\alpha}=\frac{\sin\alpha}{1+\cos\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\,\frac{\alpha}{2}=\frac{\sin\alpha}{1-\cos\alpha}=\frac{1+\cos\alpha}{\sin\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,\frac{\alpha}{2}=\sqrt{\frac{1-\cos\alpha}{1+\cos\alpha}},\quad 0 \leqslant \alpha \lt \pi, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\,\frac{\alpha}{2}=\sqrt{\frac{1+\cos\alpha}{1-\cos\alpha}},\quad 0 \lt \alpha \leqslant \pi. }[/math]
Произведения
Формулы для произведений функций двух углов:
- [math]\displaystyle{ \sin\alpha \sin\beta = \frac{\cos(\alpha-\beta) - \cos(\alpha+\beta)}{2}, }[/math]
- [math]\displaystyle{ \sin\alpha \cos\beta = \frac{\sin(\alpha-\beta) + \sin(\alpha+\beta)}{2}, }[/math]
- [math]\displaystyle{ \cos\alpha \cos\beta = \frac{\cos(\alpha-\beta) + \cos(\alpha+\beta)}{2}, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,\alpha\,\operatorname{tg}\,\beta = \frac{\cos(\alpha-\beta) - \cos(\alpha+\beta)}{\cos(\alpha-\beta) + \cos(\alpha+\beta)}, }[/math]
- [math]\displaystyle{ \operatorname{tg}\,\alpha\,\operatorname{ctg}\,\beta = \frac{\sin(\alpha-\beta) + \sin(\alpha+\beta)}{\sin(\alpha+\beta) -\sin(\alpha-\beta)}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}\,\alpha\,\operatorname{ctg}\,\beta = \frac{\cos(\alpha-\beta) + \cos(\alpha+\beta)}{\cos(\alpha-\beta) - \cos(\alpha+\beta)}. }[/math]
Аналогичные формулы для произведений синусов и косинусов трёх углов:
- [math]\displaystyle{ \sin\alpha \sin\beta \sin\gamma = \frac{\sin(\alpha+\beta-\gamma) + \sin(\beta+\gamma-\alpha) + \sin(\alpha-\beta+\gamma) - \sin(\alpha+\beta+\gamma)}{4}, }[/math]
- [math]\displaystyle{ \sin\alpha \sin\beta \cos\gamma = \frac{-\cos(\alpha+\beta-\gamma) + \cos(\beta+\gamma-\alpha) + \cos(\alpha-\beta+\gamma) - \cos(\alpha+\beta+\gamma)}{4}, }[/math]
- [math]\displaystyle{ \sin\alpha \cos\beta \cos\gamma = \frac{\sin(\alpha+\beta-\gamma) - \sin(\beta+\gamma-\alpha) + \sin(\alpha-\beta+\gamma) - \sin(\alpha+\beta+\gamma)}{4}, }[/math]
- [math]\displaystyle{ \cos\alpha \cos\beta \cos\gamma = \frac{\cos(\alpha+\beta-\gamma) + \cos(\beta+\gamma-\alpha) + \cos(\alpha-\beta+\gamma) + \cos(\alpha+\beta+\gamma)}{4}. }[/math]
Формулы для произведений тангенсов и котангенсов трёх углов можно получить, поделив правые и левые части соответствующих равенств, представленных выше.
Степени
- [math]\displaystyle{ \sin^2\alpha = \frac{1 - \cos 2\,\alpha}{2} = \frac{\operatorname{tg}^2\,\alpha}{1 + \operatorname{tg}^2\,\alpha}, }[/math]
- [math]\displaystyle{ \cos^2\alpha = \frac{1 + \cos 2\,\alpha}{2} = \frac{\operatorname{ctg}^2\,\alpha}{1 + \operatorname{ctg}^2\,\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{tg}^2\,\alpha = \frac{1 - \cos 2\,\alpha}{1 + \cos 2\,\alpha} = \frac{\operatorname{sin}^2\,\alpha}{1 - \operatorname{sin}^2\,\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}^2\,\alpha = \frac{1 + \cos 2\,\alpha}{1 - \cos 2\,\alpha} = \frac{\operatorname{cos}^2\,\alpha}{1 - \operatorname{cos}^2\,\alpha}, }[/math]
- [math]\displaystyle{ \sin^3\alpha = \frac{3\sin\alpha - \sin 3\,\alpha}{4}, }[/math]
- [math]\displaystyle{ \cos^3\alpha = \frac{3\cos\alpha + \cos 3\,\alpha}{4}, }[/math]
- [math]\displaystyle{ \operatorname{tg}^3\,\alpha = \frac{3\sin\alpha - \sin 3\,\alpha}{3\cos\alpha + \cos 3\,\alpha}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}^3\,\alpha = \frac{3\cos\alpha + \cos 3\,\alpha}{3\sin\alpha - \sin 3\,\alpha}, }[/math]
- [math]\displaystyle{ \sin^4\alpha = \frac{\cos 4\alpha - 4\cos 2\,\alpha + 3}{8}, }[/math]
- [math]\displaystyle{ \cos^4\alpha = \frac{\cos 4\alpha + 4\cos 2\,\alpha + 3}{8}, }[/math]
- [math]\displaystyle{ \operatorname{tg}^4\,\alpha = \frac{\cos 4\alpha - 4\cos 2\,\alpha + 3}{\cos 4\alpha + 4\cos 2\,\alpha + 3}, }[/math]
- [math]\displaystyle{ \operatorname{ctg}^4\,\alpha = \frac{\cos 4\alpha + 4\cos 2\,\alpha + 3}{\cos 4\alpha - 4\cos 2\,\alpha + 3}. }[/math]
Суммы
- [math]\displaystyle{ \sin \alpha \pm \sin \beta = 2 \sin \frac{\alpha \pm \beta}{2} \cos \frac{\alpha \mp \beta}{2}, }[/math]
- [math]\displaystyle{ \cos \alpha + \cos \beta = 2 \cos \frac{\alpha+\beta}{2} \cos \frac{\alpha-\beta}{2}, }[/math]
- [math]\displaystyle{ \cos \alpha - \cos \beta = - 2 \sin \frac{\alpha+\beta}{2} \sin \frac{\alpha-\beta}{2}, }[/math]
- [math]\displaystyle{ \operatorname{tg} \alpha \pm \operatorname{tg} \beta = \frac{\sin (\alpha \pm \beta)}{\cos \alpha \cos \beta}, }[/math]
- [math]\displaystyle{ \operatorname{ctg} \alpha \pm \operatorname{ctg} \beta = \frac{\sin (\beta \pm \alpha)}{\sin \alpha \sin \beta}, }[/math]
- [math]\displaystyle{ 1 \pm \sin {2 \alpha} = (\sin \alpha \pm \cos \alpha)^2, }[/math]
- [math]\displaystyle{ \sin \alpha\pm\cos \alpha=\sqrt{2}\cdot\sin\left(\alpha\pm{\pi\over4}\right) . }[/math]
Существует представление:
- [math]\displaystyle{ A \sin \alpha + B \cos \alpha = \sqrt{A^2 + B^2}\;\sin( \alpha + \phi ), }[/math]
где угол [math]\displaystyle{ \phi }[/math] находится из соотношений:
- [math]\displaystyle{ \sin \phi = \frac{B}{\sqrt{A^2 + B^2}}, }[/math]
- [math]\displaystyle{ \cos \phi = \frac{A}{\sqrt{A^2 + B^2}}. }[/math]
Универсальная тригонометрическая подстановка
Все тригонометрические функции можно выразить через тангенс половинного угла:
[math]\displaystyle{ \sin x = \frac{\sin x}{1} = \frac{2\sin \frac{x}{2}\cos \frac{x}{2}}{\sin^2 \frac{x}{2} + \cos^2 \frac{x}{2}} =\frac{2\operatorname{tg} \frac{x}{2}}{1 + \operatorname{tg}^2 \frac{x}{2}}, }[/math]
[math]\displaystyle{ \cos x = \frac{\cos x}{1} = \frac{\cos^2 \frac{x}{2} - \sin^2 \frac{x}{2}}{\cos^2 \frac{x}{2} + \sin^2 \frac{x}{2}} =\frac{1 - \operatorname{tg}^2 \frac{x}{2}}{1 + \operatorname{tg}^2 \frac{x}{2}}, }[/math]
[math]\displaystyle{ \operatorname{tg}~x = \frac{\sin x}{\cos x} = \frac{2\operatorname{tg} \frac{x}{2}}{1 - \operatorname{tg}^2 \frac{x}{2}}, }[/math]
[math]\displaystyle{ \operatorname{ctg}~x = \frac{\cos x}{\sin x} = \frac{1 - \operatorname{tg}^2 \frac{x}{2}}{2\operatorname{tg} \frac{x}{2}}, }[/math]
[math]\displaystyle{ \sec x = \frac{1}{\cos x} = \frac{1 + \operatorname{tg}^2 \frac{x}{2}}{1 - \operatorname{tg}^2 \frac{x}{2}}, }[/math]
[math]\displaystyle{ \operatorname{cosec}~x = \frac{1}{\sin x} = \frac{1 + \operatorname{tg}^2 \frac{x}{2}} {2\operatorname{tg} \frac{x}{2}}. }[/math]
Исследование функций в математическом анализе
Разложение в бесконечные произведения
Тригонометрические функции могут быть представлены в виде бесконечного произведения многочленов:
- [math]\displaystyle{ \sin x=x\,\prod_{n=1}^\infty \left(1 - \frac{x^2}{\pi ^2n^2} \right), }[/math]
- [math]\displaystyle{ \cos x = \prod_{n=0}^\infty \left( 1 - \frac{4x^2}{\pi^2(2n+1)^2} \right). }[/math]
Эти соотношения выполняются при любом значении [math]\displaystyle{ x }[/math].
Непрерывные дроби
Разложение тангенса в непрерывную дробь:
- [math]\displaystyle{ \mathop{\rm tg} x=\frac{x}{1-\frac{x^2}{3-\frac{x^2}{5-\frac{x^2}{7-\frac{x^2}{\ddots}}}}} }[/math]
Производные и первообразные
Все тригонометрические функции непрерывно и неограниченно дифференцируемы на всей области определения:
[math]\displaystyle{ ( \sin x )' = \cos x \,, }[/math]
[math]\displaystyle{ ( \cos x )' = -\sin x \,, }[/math]
[math]\displaystyle{ ( \operatorname{tg} x )' = \frac{1}{\cos ^2 x}= 1+\operatorname{tg}^2 x=\sec^2 x, }[/math]
[math]\displaystyle{ ( \operatorname{ctg} x )' = -\frac{1}{\sin ^2 x}=-\operatorname{cosec}^2 x, }[/math]
[math]\displaystyle{ ( \sec x)' = \frac{\sin x}{\cos ^2 x}=\sec x \operatorname{tg} x, }[/math]
[math]\displaystyle{ ( \operatorname{cosec}~x)' = -\frac{\cos x}{\sin ^2 x}. }[/math]
Интегралы тригонометрических функций на области определения выражаются через элементарные функции следующим образом[6]:
[math]\displaystyle{ \int\sin x\, dx = -\cos x + C \,, }[/math]
[math]\displaystyle{ \int\cos x\, dx = \sin x + C \,, }[/math]
[math]\displaystyle{ \int\operatorname{tg} x\, dx = -\ln \left| \cos x\right| + C \,, }[/math]
[math]\displaystyle{ \int\operatorname{ctg} x\, dx = \ln \left| \sin x \right| + C \,, }[/math]
[math]\displaystyle{ \int\sec x\, dx=\ln \left| \operatorname{tg} \, \left( \frac {\pi}{4}+\frac{x}{2}\right) \right|+ C \,, }[/math]
[math]\displaystyle{ \int \operatorname{cosec}~ x\, dx=\ln \left| \operatorname{tg} \, \frac{x}{2} \right|+ C. }[/math]
Тригонометрические функции комплексного аргумента
Определение
- [math]\displaystyle{ e^{i \vartheta} = \cos\vartheta + i\sin\vartheta. }[/math]
Формула Эйлера позволяет определить тригонометрические функции от комплексных аргументов через экспоненту по аналогии с гиперболическими функциями, или (с помощью рядов) как аналитическое продолжение их вещественных аналогов:
- [math]\displaystyle{ \sin z = \sum_{n=0}^\infty \frac{(-1)^{n}}{(2n+1)!}z^{2n+1} = \frac{e^{i z} - e^{-i z}}{2i}\, = \frac{\operatorname{sh} i z }{i}; }[/math]
- [math]\displaystyle{ \cos z = \sum_{n=0}^\infty \frac{(-1)^{n}}{(2n)!}z^{2n} = \frac{e^{i z} + e^{-i z}}{2}\, = \operatorname{ch} i z; }[/math]
- [math]\displaystyle{ \operatorname{tg}\, z = \frac{\sin z}{\cos z} = \frac{e^{i z} - e^{-i z}}{i(e^{i z} + e^{-i z})}; }[/math]
- [math]\displaystyle{ \operatorname{ctg}\, z = \frac{\cos z}{\sin z} = \frac{i(e^{i z} + e^{-i z})}{e^{i z} - e^{-i z}}; }[/math]
- [math]\displaystyle{ \sec z = \frac{1}{\cos z} = \frac{2}{e^{i z} + e^{-i z}}; }[/math]
- [math]\displaystyle{ \operatorname{cosec}\, z = \frac{1}{\sin z} = \frac{2i}{e^{i z} - e^{-i z}}, }[/math] где [math]\displaystyle{ i^2=-1. }[/math]
Соответственно, для вещественного x:
- [math]\displaystyle{ \cos x = \operatorname{Re}(e^{i x}), }[/math]
- [math]\displaystyle{ \sin x = \operatorname{Im}(e^{i x}). }[/math]
Комплексные синус и косинус тесно связаны с гиперболическими функциями:
- [math]\displaystyle{ \sin (x + iy) = \sin x\, \operatorname{ch}\, y + i \cos x\, \operatorname{sh}\, y, }[/math]
- [math]\displaystyle{ \cos (x + iy) = \cos x\, \operatorname{ch}\, y - i \sin x\, \operatorname{sh}\, y. }[/math]
Большинство перечисленных выше свойств тригонометрических функций сохраняются и в комплексном случае. Некоторые дополнительные свойства:
- комплексные синус и косинус, в отличие от вещественных, могут принимать сколь угодно большие по модулю значения;
- все нули комплексных синуса и косинуса лежат на вещественной оси.
Комплексные графики
На следующих графиках изображена комплексная плоскость, а значения функций выделены цветом. Яркость отражает абсолютное значение (чёрный — ноль). Цвет изменяется от аргумента и угла согласно карте.
История названий
Линия синуса (линия [math]\displaystyle{ AB }[/math] на рис. 2) у индийских математиков первоначально называлась «арха-джива» («полутетива», то есть половина хорды данной дуги, поскольку дуга с хордой напоминает лук с тетивой). Затем слово «арха» было отброшено и линию синуса стали называть просто «джива». Арабские математики, переводя индийские книги с санскрита, не перевели слово «джива» арабским словом «ватар», обозначающим тетиву и хорду, а транскрибировали его арабскими буквами и стали называть линию синуса «джиба» (جيب). Так как в арабском языке краткие гласные не обозначаются, а долгое «и» в слове «джиба» обозначается так же, как полугласная «й», арабы стали произносить название линии синуса как «джайб», что буквально обозначает «впадина», «пазуха». При переводе арабских сочинений на латынь европейские переводчики перевели слово «джайб» латинским словом sinus — «синус», имеющим то же значение (именно в этом значении оно применяется как анатомический термин синус). Термин «косинус» (лат. cosinus) — это сокращение от лат. complementi sinus — дополнительный синус.
Современные краткие обозначения [math]\displaystyle{ \sin }[/math], [math]\displaystyle{ \cos }[/math] введены Уильямом Отредом и Бонавентурой Кавальери и закреплены в трудах Леонарда Эйлера.
Термины «тангенс» (лат. tangens — касающийся) и «секанс» (лат. secans — секущий) были введены датским математиком Томасом Финке в его книге «Геометрия круглого» (Geometria rotundi, 1583).
Сам термин тригонометрические функции введён Клюгелем в 1770 году.
Позднее были введены и термины для обратных тригонометрических функций — арксинус, арккосинус, арктангенс, арккотангенс, арксеканс, арккосеканс — с помощью добавления приставки «арк» (от лат. arcus — дуга), — Ж. Лагранжем и др.
См. также
- Гиперболические функции
- Интегральный синус
- Интегральный косинус
- Интегральный секанс
- Обратные тригонометрические функции
- Редко используемые тригонометрические функции
- Решение треугольников
- Синус-верзус
- Сферическая тригонометрия
- Тригонометрические тождества
- Тригонометрические функции от матрицы
- Тригонометрический ряд Фурье
- Функция Гудермана
- Четырёхзначные математические таблицы (Таблицы Брадиса)
- Эллиптические функции
Литература
- Бермант А. Ф., Люстерник Л. А. Тригонометрия. — М.: Наука, 1967.
- Тригонометрические функции — статья из Большой советской энциклопедии. — М.: Советская энциклопедия, 1977. — Т. 26. — С. 204—206.
- Бронштейн И. Н., Семендяев К. А. Прямолинейная тригонометрия // Справочник по математике. — Изд. 7-е, стереотипное. — М.: Государственное издательство технико-теоретической литературы, 1967. — С. 179—184.
- Выгодский М. Я. Справочник по элементарной математике. — М.: Наука, 1978.
- Переиздание: М.: АСТ, 2006. — 509 с. — ISBN 5-17-009554-6 www.alleng.ru/d/math/math42.htm
- Двайт Г. Б. Тригонометрические функции // Таблицы интегралов и другие математические формулы. — 4-е изд. — М.: Наука, 1973. — С. 70—102.
- Кожеуров П. А. Тригонометрия. — М.: Физматгиз, 1963.
- Маркушевич А. И. Замечательные синусы. — М.: Наука, 1974.
- Математическая энциклопедия / Гл. ред. И. М. Виноградов. — М.: Советская энциклопедия, 1984. — И. М. Виноградов. Тригонометрические функции // Математическая энциклопедия. — М.: Советская энциклопедия. — 1977—1985.
- Тригонометрические функции // Энциклопедический словарь юного математика / Ред. коллегия, Гнеденко Б. В. (гл. ред.), Савин А. П. и др. — М.: Педагогика, 1985 (1989). — С. 299—301—305. — 352 с., ил. — ISBN 5-7155-0218-7 (С. 342, 343 — таблицы тригонометрических функций 0°-90°, в том числе в радианах)
- Тригонометрические функции // Справочник по математике (для ср. уч. заведений) / Цыпкин А. Г., под ред. Степанова С. А. — 3-е изд. — М.: Наука, Гл. редакция физ.-мат. литературы, 1983. — С. 240—258. — 480 с.
Ссылки
- GonioLab — прояснённая единичная окружность, тригонометрические и гиперболические функции (Java Web Start)
- Weisstein, Eric W. Trigonometric Functions (англ.) на сайте Wolfram MathWorld.
- Онлайн калькулятор: вычисление значений тригонометрических функций (в том числе нахождение углов треугольника по сторонам)
- Интерактивная карта значений тригонометрических функций
- Тригонометрические таблицы (0° — 360°)
- «Синус и косинус — это проценты» — перевод статьи How To Learn Trigonometry Intuitively | BetterExplained (англ.)
Примечания
- ↑ Справочник: Корн Г., Корн Т. Справочник по математике (для научных работников и инженеров). — М.: Наука, 1973. — 720 с. Архивная копия от 19 января 2015 на Wayback Machine относит их к специальным функциям.
- ↑ Знак математический. // Большая советская энциклопедия. 1-е изд. Т. 27. — М., 1933.
- ↑ Справочник по элементарной математике, 1978, с. 271—272.
- ↑ Справочник по элементарной математике, 1978, с. 282—284.
- ↑ Ильин В. А., Позняк Э. Г. Основы математического анализа. Ч. 1. — М.: Наука, 1998. — ISBN 5-02-015231-5.
- ↑ В формулах, содержащих логарифм в правой части равенств, константы интегрирования [math]\displaystyle{ \scriptstyle C }[/math], вообще говоря, различны для различных интервалов непрерывности.





