Замечательные пределы

Замеча́тельные преде́лы — термины, использующиеся в советских и российских учебниках по математическому анализу для обозначения двух широко известных математических тождеств со взятием предела:

• Первый замечательный предел:

  [math]\displaystyle{ \lim_{x \to 0}\frac{\sin x}{x} = 1. }[/math]

• Второй замечательный предел:

  [math]\displaystyle{ \lim_{x \to \infty}\left(1 + \frac{1}{x}\right)^x = e. }[/math]

Первый замечательный предел

[math]\displaystyle{ \lim_{x \to 0}\frac{\sin x}{x} = 1 }[/math]

Доказательство:

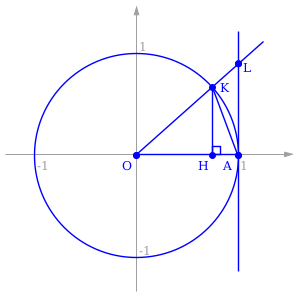
Рассмотрим односторонние пределы [math]\displaystyle{ \lim_{x \to +0}\frac{\sin x}{x} }[/math] и [math]\displaystyle{ \lim_{x \to {\displaystyle-}0}\frac{\sin x}{x} }[/math] и докажем, что они равны 1.

Рассмотрим случай [math]\displaystyle{ x \in \left( 0; \frac{\pi}{2} \right) }[/math]. Отложим этот угол на единичной окружности так, чтобы его вершина совпадала с началом координат, а одна сторона совпадала с осью [math]\displaystyle{ OX }[/math]. Пусть [math]\displaystyle{ K }[/math] — точка пересечения второй стороны угла с единичной окружностью, а точка [math]\displaystyle{ L }[/math] — с касательной к этой окружности в точке [math]\displaystyle{ A=\left( 1; 0 \right) }[/math]. Точка [math]\displaystyle{ H }[/math] — проекция точки [math]\displaystyle{ K }[/math] на ось [math]\displaystyle{ OX }[/math].

Очевидно, что:

[math]\displaystyle{ S_{\triangle OKA} \lt S_{sect KOA} \lt S_{\triangle OAL} }[/math] (1)

(где [math]\displaystyle{ S_{sect KOA} }[/math] — площадь сектора [math]\displaystyle{ KOA }[/math])

Поскольку [math]\displaystyle{ \left| KH \right| = \sin x, \, \left| LA \right| = \operatorname{tg} x }[/math]:

[math]\displaystyle{ S_{\triangle OKA} = \frac{1}{2} \cdot \left| OA \right| \cdot \left| KH \right| = \frac{1}{2} \cdot 1 \cdot \sin x = \frac{\sin x}{2} }[/math]

[math]\displaystyle{ S_{sect KOA} = \frac{1}{2} \cdot \left| OA \right| ^2 \cdot x = \frac{x}{2} }[/math]

[math]\displaystyle{ S_{\triangle OAL} = \frac{1}{2} \cdot \left| OA \right| \cdot \left| LA \right| = \frac{\operatorname{tg} x}{2} }[/math]

Подставляя в (1), получим:

[math]\displaystyle{ \frac{\sin x}{2} \lt \frac{x}{2} \lt \frac{\operatorname{tg} x}{2} }[/math]

Так как при [math]\displaystyle{ x \to +0: \sin x \gt 0, \, x \gt 0, \, \operatorname{tg} x \gt 0 }[/math]:

[math]\displaystyle{ \frac{1}{\operatorname{tg} x} \lt \frac{1}{x} \lt \frac{1}{\sin x} }[/math]

Умножаем на [math]\displaystyle{ \sin x }[/math]:

[math]\displaystyle{ \cos x \lt \frac{\sin x}{x} \lt 1 }[/math]

Перейдём к пределу:

[math]\displaystyle{ \lim_{x \to +0}\cos x \leqslant \lim_{x \to +0}\frac{\sin x}{x} \leqslant 1 }[/math]

[math]\displaystyle{ 1 \leqslant \lim_{x \to +0}\frac{\sin x}{x} \leqslant 1 }[/math]

[math]\displaystyle{ \lim_{x \to +0} \frac{\sin x}{x} = 1 }[/math]

Найдём левый односторонний предел (так как функция четна, в этом нет необходимости, достаточно доказать это для правого предела):

[math]\displaystyle{ \lim_{x \to -0}\frac{\sin x}{x} = \left[ \begin{matrix} u = -x \\ x = -u \\ u \to +0 \\ x \to -0 \end{matrix} \right] = \lim_{u \to +0}\frac{\sin(-u)}{-u} = \lim_{u \to +0}\frac{-\sin(u)}{-u} = \lim_{u \to +0}\frac{\sin(u)}{u} = 1 }[/math]

Правый и левый односторонний пределы существуют и равны 1, а значит и сам предел равен 1.

Следствия:

• [math]\displaystyle{ \lim_{x \to 0}\frac{\operatorname{tg} x}{x} = 1 }[/math]
• [math]\displaystyle{ \lim_{x \to 0}\frac{\operatorname{arcsin} x}{x} = 1 }[/math]
• [math]\displaystyle{ \lim_{x \to 0}\frac{\operatorname{arctg} x}{x} = 1 }[/math]
• [math]\displaystyle{ \lim_{x \to 0}\frac{1 - \cos x}{ \frac{x^2}{2} } = 1 }[/math]

Доказательство следствий

[math]\displaystyle{ \lim_{x \to 0}\frac{\operatorname{tg} x}{x} = \lim_{x \to 0}\frac{\sin x}{x \cos x} = \lim_{x \to 0}\frac{\sin x}{x} \cdot \lim_{x \to 0}\frac{1}{\cos x} = 1 \cdot 1 = 1 }[/math]

[math]\displaystyle{ \lim_{x \to 0}\frac{\operatorname{arcsin} x}{x} = \left [ \begin{matrix} u = \operatorname{arcsin} x \\ x = \sin u \\ u \to 0 \\ x \to 0 \end{matrix} \right ] = \lim_{u \to 0}\frac{u}{\sin u} = 1 }[/math]

[math]\displaystyle{ \lim_{x \to 0}\frac{\operatorname{arctg} x}{x} = \left [ \begin{matrix} u = \operatorname{arctg} x \\ x = \operatorname{tg} u \\ u \to 0 \\ x \to 0 \end{matrix} \right ] = \lim_{u \to 0}\frac{u}{\operatorname{tg} u} = 1 }[/math]

[math]\displaystyle{ \lim_{x \to 0}\frac{1 - \cos x}{\frac{x^2}{2} } = \lim_{x \to 0}\frac{2 \cdot \sin^2 \frac{x}{2}}{2 \cdot \left( \frac{x}{2} \right) ^2} = \lim_{\frac{x}{2} \to 0}\left( \frac{\sin \frac{x}{2}}{\frac{x}{2}} \right) ^2 = 1^2 = 1 }[/math]

Второй замечательный предел

[math]\displaystyle{ \lim_{x \to \infty}\left(1 + \frac{1}{x}\right)^x = e }[/math] или [math]\displaystyle{ \lim_{x \to 0}\left(1 + x\right)^{1/x} = e }[/math]

Доказательство существования второго замечательного предела:

Доказательство для натуральных значений x

[math]\displaystyle{ \blacktriangleleft }[/math]  Докажем вначале теорему для случая последовательности [math]\displaystyle{ x_{n} = \left(1 + \frac{1}{n}\right)^n;\ n\in\mathbb N }[/math]

По формуле бинома Ньютона: [math]\displaystyle{ (a + b)^n = a^n~+~\frac{n}{1}\cdot a^{n-1}\cdot b~+~\frac{n(n-1)}{1\cdot 2}\cdot a^{n-2}\cdot b^2 ~+~ ... ~+~ \frac{n(n-1)(n-2)...(n-(n-1))}{1\cdot 2\cdot 3 \cdot ... \cdot n}\cdot b^n;\ n\in\mathbb N }[/math]

Полагая [math]\displaystyle{ a=1;~b=\frac{1}{n} }[/math], получим:

[math]\displaystyle{ \left(1 + \frac{1}{n}\right)^n = 1~+~\frac{n}{1}\cdot\frac{1}{n}~+~\frac{n(n-1)}{1\cdot2}\cdot \frac{1}{n^2}~+~\frac{n(n-1)(n-2)}{1\cdot2\cdot3}\cdot\frac{1}{n^3}~+~...~+~\frac{n(n-1)(n-2)...(n-(n-1))}{1\cdot2\cdot3\cdot...\cdot n}\cdot \frac{1}{n^n} = }[/math]

[math]\displaystyle{ =1~+~1~+~\frac{1}{1\cdot2}\cdot\left(1-\frac{1}{n}\right)~+~\frac{1}{1\cdot2\cdot3}\cdot\left(1-\frac{1}{n}\right)\cdot\left(1-\frac{2}{n}\right)~+~ ... ~+~ \frac{1}{1\cdot2\cdot3\cdot...\cdot n}\cdot\left(1-\frac{1}{n}\right)\cdot\left(1-\frac{2}{n}\right)\cdot...\cdot\left(1-\frac{n-1}{n}\right) }[/math]       (1)

С увеличением [math]\displaystyle{ n }[/math] число положительных слагаемых в правой части равенства (1) увеличивается. Кроме того, при увеличении [math]\displaystyle{ n }[/math] число [math]\displaystyle{ \frac{1}{n} }[/math] убывает, поэтому величины [math]\displaystyle{ \left(1 - \frac{1}{n}\right), \left(1-\frac{2}{n}\right), ... }[/math] возрастают. Поэтому последовательность [math]\displaystyle{ \{x_{n}\} = \left\{\left(1 + \frac{1}{n}\right)^n\right\};\ n\in\mathbb N }[/math] — возрастающая, при этом

[math]\displaystyle{ \left(1 + \frac{1}{n}\right)^n \ge 2, n\in\mathbb N }[/math]      (2).

Покажем, что она ограничена. Заменим каждую скобку в правой части равенства на единицу, правая часть увеличится, получим неравенство

[math]\displaystyle{ \left(1+\frac{1}{n}\right)^n \lt 1+1+\frac{1}{1\cdot2}+\frac{1}{1\cdot2\cdot3}~+~...~+~\frac{1}{1\cdot2\cdot3\cdot ... \cdot n} }[/math]

Усилим полученное неравенство, заменим 3,4,5, …, стоящие в знаменателях дробей, числом 2:

[math]\displaystyle{ \left(1+\frac{1}{n}\right)^n\lt 1+\left(1+\frac{1}{2}+\frac{1}{2^2}+...+\frac{1}{2^{n-1}}\right) }[/math].

Сумму в скобке найдём по формуле суммы членов геометрической прогрессии:

[math]\displaystyle{ 1+\frac{1}{2}+\frac{1}{2^2}+...+\frac{1}{2^{n-1}} = \frac{1\cdot\left(1-(\frac{1}{2})^n\right)}{1-\frac{1}{2}}=2\cdot \left(1-\frac{1}{2^n}\right)\lt 2 }[/math].

Поэтому [math]\displaystyle{ \left(1+\frac{1}{n}\right)^n\lt 1+2=3 }[/math]      (3).

Итак, последовательность ограничена сверху, при этом [math]\displaystyle{ \forall n\in\mathbb N }[/math] выполняются неравенства (2) и (3):   [math]\displaystyle{ 2 \le \left(1+\frac{1}{n}\right)^n \lt 3 }[/math].

Следовательно, на основании теоремы Вейерштрасса (критерий сходимости последовательности) последовательность [math]\displaystyle{ x_{n} = \left(1 + \frac{1}{n}\right)^n,\ n\in\mathbb N }[/math] монотонно возрастает и ограничена, значит имеет предел, обозначаемый буквой e. Т.е. [math]\displaystyle{ \lim_{n \to \infty}\left(1 + \frac{1}{n}\right)^n = e }[/math] [math]\displaystyle{ \blacktriangleright }[/math]

[math]\displaystyle{ \blacktriangleleft }[/math]   Зная, что второй замечательный предел верен для натуральных значений x, докажем второй замечательный предел для вещественных x, то есть докажем, что [math]\displaystyle{ \lim_{x \to \infty}\left(1 + \frac{1}{x}\right)^x = e;\ x\in\mathbb R }[/math]. Рассмотрим два случая:

1. Пусть [math]\displaystyle{ x \rightarrow +\infty }[/math]. Каждое значение x заключено между двумя положительными целыми числами: [math]\displaystyle{ n\leqslant x\lt n+1 }[/math], где [math]\displaystyle{ n = [x] }[/math] — это целая часть x.

Отсюда следует: [math]\displaystyle{ \frac{1}{n+1}\lt \frac{1}{x}\leqslant \frac{1}{n}~~\Longleftrightarrow~~1+\frac{1}{n+1}\lt 1+\frac{1}{x}\leqslant 1+\frac{1}{n} }[/math], поэтому

[math]\displaystyle{ \left(1+\frac{1}{n+1}\right)^n\lt \left(1+\frac{1}{x}\right)^x\leqslant\left(1+\frac{1}{n}\right)^{n+1} }[/math].

Если [math]\displaystyle{ x \rightarrow +\infty }[/math], то [math]\displaystyle{ n \rightarrow \infty }[/math]. Поэтому, согласно пределу [math]\displaystyle{ \lim_{n \to \infty}\left(1 + \frac{1}{n}\right)^n = e }[/math], имеем:

[math]\displaystyle{ \lim_{n \to \infty}\left(1 + \frac{1}{n+1}\right)^n = \frac{\lim\limits_{n \to \infty}(1 + \frac{1}{n+1})^{n+1}}{\lim\limits_{n \to \infty}\left(1 + \frac{1}{n+1}\right)} = \frac{e}{1}=e }[/math]

[math]\displaystyle{ \lim_{n \to \infty}\left(1 + \frac{1}{n}\right)^{n+1} = \lim_{n \to \infty}\left(1 + \frac{1}{n}\right)^n\cdot \lim_{n \to \infty}\left(1 + \frac{1}{n}\right)=e\cdot 1=e }[/math].

По признаку (о пределе промежуточной функции) существования пределов [math]\displaystyle{ \lim_{x \to +\infty}\left(1 + \frac{1}{x}\right)^x = e }[/math].

2. Пусть [math]\displaystyle{ x \to -\infty }[/math]. Сделаем подстановку [math]\displaystyle{ - x = t }[/math], тогда

[math]\displaystyle{ \lim_{x \to -\infty}\left(1 + \frac{1}{x}\right)^x = \lim_{t \to +\infty}\left(1 - \frac{1}{t}\right)^{-t}= \lim_{t \to +\infty}\left(\frac{t}{t-1}\right)^t = \lim_{t \to +\infty}\left(1 + \frac{1}{t-1}\right)^t = }[/math]

[math]\displaystyle{ = \lim_{t \to +\infty}\left(1 + \frac{1}{t-1}\right)^{t-1}\cdot \lim_{t \to +\infty}\left(1 + \frac{1}{t-1}\right)^1 = e\cdot1=e }[/math].

Очевидно, из двух этих случаев вытекает, что [math]\displaystyle{ \lim_{x \to \infty}\left(1 + \frac{1}{x}\right)^x = e }[/math] для вещественного x.    [math]\displaystyle{ \blacktriangleright }[/math]

Следствия

1. [math]\displaystyle{ \lim_{u \to 0}(1 + u)^\frac{1}{u}=e }[/math]
2. [math]\displaystyle{ \lim_{x \to \infty}\left(1 + \frac{k}{x}\right)^x=e^k }[/math]
3. [math]\displaystyle{ \lim_{x \to 0}\frac{\ln(1 + x)}{x} = 1 }[/math]
4. [math]\displaystyle{ \lim_{x \to 0}\frac{e^x - 1}{x} = 1 }[/math]
5. [math]\displaystyle{ \lim_{x \to 0}\frac{a^x - 1}{x \ln a} = 1 }[/math] для [math]\displaystyle{ a \gt 0 }[/math], [math]\displaystyle{ a \neq 1 }[/math]
6. [math]\displaystyle{ \lim_{x \to 0}\frac{(1 + x)^\alpha - 1}{\alpha x} = 1 }[/math]
7. [math]\displaystyle{ \lim_{x \to \infty}\left(1 - \frac{k}{x}\right)^x=e^{-k} }[/math]

Доказательства следствий

1. [math]\displaystyle{ \lim_{u \to 0}(1 + u)^\frac{1}{u} = \left [ \begin{matrix} u = 1/x \\ x \to \infty \end{matrix} \right ] =\lim_{x \to \infty}\left(1 + \frac{1}{x}\right)^x=e }[/math]
2. [math]\displaystyle{ \lim_{x \to \infty}\left(1 + \frac{k}{x}\right)^x = \left [ \begin{matrix} u = x/k \\ x = k u \\ u \to \infty \\ x \to \infty \end{matrix} \right ] = \lim_{u \to \infty}\left(1 + \frac{1}{u}\right)^{ku} = \left(\lim_{u \to \infty}\left(1 + \frac{1}{u}\right)^u\right)^k = e^k }[/math]
3. [math]\displaystyle{ \lim_{x \to 0}\frac{\ln(1 + x)}{x} = \lim_{x \to 0}\frac{1}{x}\ln(1 + x) = \lim_{x \to 0}\ln((1 + x)^\frac{1}{x}) = \ln e = 1 }[/math]
4. [math]\displaystyle{ \lim_{x \to 0}\frac{e^x - 1}{x} = \left [ \begin{matrix} u = e^x - 1 \\ x = \ln(1 + u) \\ x \to 0 \\ u \to 0 \end{matrix} \right ] = \lim_{u \to 0}\frac{u}{\ln(1 + u)} = 1 }[/math]
5. [math]\displaystyle{ \lim_{x \to 0}\frac{a^x - 1}{x \ln a} = \lim_{x \to 0}\frac{e^{\ln(a^x)} - 1}{x \ln a} = \lim_{x \to 0}\frac{e^{x \ln a} - 1}{x \ln a} = \left [ \begin{matrix} u = x \ln a \\ u \to 0 \\ x \to 0 \end{matrix} \right ] = \lim_{u \to 0}\frac{e^u - 1}{u} = 1 }[/math]
6. [math]\displaystyle{ \lim_{x \to 0}\frac{(1 + x)^\alpha - 1}{\alpha x} = \lim_{x \to 0}\frac{e^{\alpha \ln(1+x)} - 1}{\alpha x} = \lim_{x \to 0}\frac{e^{\alpha \ln(1+x)} - 1}{\alpha \ln(1+x)} \cdot \lim_{x \to 0}\frac{\ln(1+x)}{x} = }[/math]

[math]\displaystyle{ =\lim_{x \to 0}\frac{e^{\alpha \ln(1+x)} - 1}{\alpha \ln(1+x)}\cdot 1 = \left [ \begin{matrix} u = \alpha \ln (1+x) \\ x \to 0 \\ u \to 0 \end{matrix} \right ]=\lim_{u \to 0}\frac{e^u - 1}{u} = 1 }[/math]

Применение

Замечательные пределы и их следствия используются при раскрытии неопределённостей для нахождения других пределов.

См. также

• Список пределов

Литература

• Ильин В. А., Позняк Э. Г. Основы математического анализа (в двух частях). — М.: Физматлит, 2005. — С. 24-25. — ISBN 5-9221-0536-1.

Ссылки

• Замечательные пределы на Wikia science Математика Архивная копия от 22 сентября 2018 на Wayback Machine