Обратные тригонометрические функции

Обра́тные тригонометри́ческие фу́нкции (круговые функции, аркфункции) — математические функции, являющиеся обратными к тригонометрическим функциям. К обратным тригонометрическим функциям обычно относят шесть функций:

• арксинус (обозначение: [math]\displaystyle{ \arcsin x; }[/math] угол, синус которого равен [math]\displaystyle{ x }[/math])
• арккосинус (обозначение: [math]\displaystyle{ \arccos x; }[/math] угол, косинус которого равен [math]\displaystyle{ x }[/math] и т. д.)
• арктангенс (обозначение: [math]\displaystyle{ \operatorname{arctg} x }[/math]; в иностранной литературе [math]\displaystyle{ \arctan x }[/math])
• арккотангенс (обозначение: [math]\displaystyle{ \operatorname{arcctg} x }[/math]; в иностранной литературе [math]\displaystyle{ \arccot x }[/math] или [math]\displaystyle{ \operatorname{arccotan} x }[/math])
• арксеканс (обозначение: [math]\displaystyle{ \arcsec x }[/math])
• арккосеканс (обозначение: [math]\displaystyle{ \operatorname{arccosec} x }[/math]; в иностранной литературе [math]\displaystyle{ \arccsc x }[/math])

Название обратной тригонометрической функции образуется от названия соответствующей ей тригонометрической функции добавлением приставки «арк-» (от лат. arcus — дуга). Это связано с тем, что геометрически значение обратной тригонометрической функции можно связать с длиной дуги единичной окружности (или углом, стягивающим эту дугу), соответствующей тому или иному отрезку. Так, обычный синус позволяет по дуге окружности найти стягивающую её хорду, а обратная функция решает противоположную задачу. Манера обозначать таким образом обратные тригонометрических функции появилась у австрийского математика XVIII века Карла Шерфера и закрепилась благодаря Лагранжу. Впервые специальный символ для обратной тригонометрической функции использовал Даниил Бернулли в 1729 году. Английская и немецкая математические школы до конца XIX века предлагали иные обозначения: [math]\displaystyle{ \sin^{-1}, \frac{1}{\sin}, }[/math] но они не прижились. Лишь изредка в иностранной литературе, также как и в научных/инженерных калькуляторах, пользуются обозначениями типа sin⁻¹, cos⁻¹ для арксинуса, арккосинуса и т. п., — такая запись считается не очень удобной, так как возможна путаница с возведением функции в степень −1.

Тригонометрические функции периодичны, поэтому функции, обратные к ним, многозначны. То есть, значение аркфункции представляет собой множество углов (дуг), для которых соответствующая прямая тригонометрическая функция равна заданному числу. Например, [math]\displaystyle{ \arcsin 1/2 }[/math] означает множество углов [math]\displaystyle{ \left ( \frac{\pi}{6}, \frac{5 \pi}{6}, \frac{13 \pi}{6}, \frac{17 \pi}{6} \dots ~ (30^\circ, 150^\circ, 390^\circ, 510^\circ \dots) \right ) }[/math], синус которых равен [math]\displaystyle{ 1/2 }[/math]. Из множества значений каждой аркфункции выделяют её главные значения (см. графики главных значений аркфункций ниже), которые обычно и имеют в виду, говоря об арксинусе, арккосинусе и т. д.

В общем случае при условии [math]\displaystyle{ -1 \leqslant \alpha \leqslant 1 }[/math] все решения уравнения [math]\displaystyle{ \sin x = \alpha }[/math] можно представить в виде [math]\displaystyle{ x = (-1)^n \arcsin \alpha + \pi n, ~ n = 0, \pm1, \pm2, \dots ~ . }[/math]

Основное соотношение

[math]\displaystyle{ \arcsin x + \arccos x = \frac{\pi}{2} }[/math]

[math]\displaystyle{ \operatorname {arctg}\, x + \operatorname {arcctg}\, x = \frac{\pi}{2} }[/math]

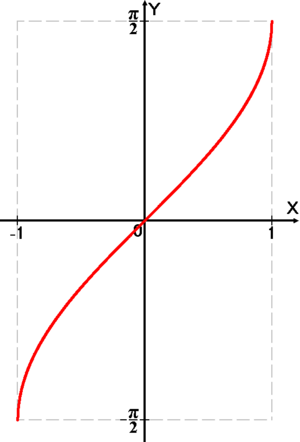
Функция arcsin

График функции [math]\displaystyle{ y = \arcsin x }[/math]

Аркси́нусом числа x называется такое значение угла y, выраженного в радианах, для которого [math]\displaystyle{ \sin y = x,\quad -\frac{\pi}{2} \leqslant y \leqslant \frac{\pi}{2},\quad |x|\leqslant 1. }[/math]

Функция [math]\displaystyle{ y=\arcsin x }[/math] непрерывна и ограничена на всей своей области определения. Она является строго возрастающей.

• [math]\displaystyle{ \sin (\arcsin x) = x\qquad }[/math] при [math]\displaystyle{ -1 \leqslant x \leqslant 1, }[/math]
• [math]\displaystyle{ \arcsin(\sin y) = y\qquad }[/math] при [math]\displaystyle{ -\frac{\pi}{2} \leqslant y \leqslant \frac{\pi}{2}, }[/math]
• [math]\displaystyle{ D(\arcsin x)=[-1; 1]\qquad }[/math] (область определения),
• [math]\displaystyle{ E(\arcsin x) = \left[-\frac{\pi}{2}; \frac{\pi}{2}\right]\qquad }[/math] (область значений).

Свойства функции arcsin

• [math]\displaystyle{ \arcsin (-x) = -\arcsin x \qquad }[/math] (функция является нечётной).
• [math]\displaystyle{ \arcsin x\gt 0 }[/math] при [math]\displaystyle{ 0 \lt x \leqslant 1 }[/math].
• [math]\displaystyle{ \arcsin x = 0 }[/math] при [math]\displaystyle{ x=0. }[/math]
• [math]\displaystyle{ \arcsin x \lt 0 }[/math] при [math]\displaystyle{ -1 \leqslant x \lt 0. }[/math]
• [math]\displaystyle{ \arcsin x = \frac{\pi}{2} - \arccos x. }[/math]
• [math]\displaystyle{ \arcsin x = \left\{\begin{matrix} \arccos \sqrt{1-x^2},\qquad 0 \leqslant x \leqslant 1 \\ -\arccos \sqrt{1-x^2},\qquad -1 \leqslant x \leqslant 0 \end{matrix}\right. }[/math]
• [math]\displaystyle{ \arcsin x = \operatorname{arctg} \frac{x}{\sqrt{1-x^2}} }[/math]
• [math]\displaystyle{ \arcsin x = \left\{\begin{matrix} \operatorname{arcctg}\, \frac{\sqrt{1-x^2}}{x},\qquad 0 \lt x \leqslant 1 \\ \operatorname{arcctg}\, \frac{\sqrt{1-x^2}}{x}-\pi,\qquad -1 \leqslant x \lt 0 \end{matrix}\right. }[/math]

Получение функции arcsin

Дана функция [math]\displaystyle{ y=\sin x }[/math]. На всей своей области определения она является кусочно-монотонной, и, значит, на всей числовой прямой обратное соответствие [math]\displaystyle{ y= \arcsin x }[/math] функцией не является. Поэтому рассмотрим отрезок [math]\displaystyle{ [-\pi/2; \pi/2] }[/math], на котором функция [math]\displaystyle{ y=\sin x }[/math] строго монотонно возрастает и принимает все значения своей области значений только один раз. Тогда на отрезке [math]\displaystyle{ [-\pi/2; \pi/2] }[/math] существует обратная функция [math]\displaystyle{ y=\arcsin x }[/math], график которой симметричен графику функции [math]\displaystyle{ y=\sin x }[/math] относительно прямой [math]\displaystyle{ y=x }[/math].

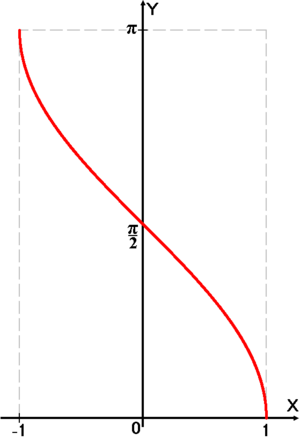
Функция arccos

График функции [math]\displaystyle{ y=\arccos x }[/math]

Аркко́синусом числа x называется такое значение угла y в радианной мере, для которого [math]\displaystyle{ \cos y = x,\qquad 0 \leqslant y \leqslant \pi,\quad |x|\leqslant 1. }[/math]

Функция [math]\displaystyle{ y=\arccos x }[/math] непрерывна и ограничена на всей своей области определения. Она является строго убывающей и неотрицательной.

• [math]\displaystyle{ \cos (\arccos x)=x }[/math] при [math]\displaystyle{ -1 \leqslant x \leqslant 1, }[/math]
• [math]\displaystyle{ \arccos (\cos y) = y }[/math] при [math]\displaystyle{ 0 \leqslant y \leqslant \pi. }[/math]
• [math]\displaystyle{ D(\arccos x)=[-1; 1] }[/math] (область определения),
• [math]\displaystyle{ E(\arccos x)=[0; \pi] }[/math] (область значений).

Свойства функции arccos

• [math]\displaystyle{ \arccos(-x) = \pi - \arccos x. }[/math] Функция центрально-симметрична относительно точки [math]\displaystyle{ \left (0; \frac{\pi}{2}\right), }[/math] является индифферентной (ни чётной, ни нечётной).
• [math]\displaystyle{ \arccos x \gt 0 }[/math] при [math]\displaystyle{ -1 \leqslant x \lt 1. }[/math]
• [math]\displaystyle{ \arccos x = 0 }[/math] при [math]\displaystyle{ x=1. }[/math]
• [math]\displaystyle{ \arccos x = \frac{\pi}{2} - \arcsin x. }[/math]
• [math]\displaystyle{ \arccos x = \left\{\begin{matrix} \arcsin \sqrt{1-x^2},\qquad 0 \leqslant x \leqslant 1 \\\pi-\arcsin \sqrt{1-x^2},\qquad -1 \leqslant x \leqslant 0 \end{matrix}\right. }[/math]
• [math]\displaystyle{ \arccos x = \operatorname{arcctg} \frac{x}{\sqrt{1-x^2}} }[/math]
• [math]\displaystyle{ \arccos x = \left\{\begin{matrix} \operatorname{arctg}\, \frac{\sqrt{1-x^2}}{x},\qquad 0 \lt x \leqslant 1 \\\pi+\operatorname{arctg}\, \frac{\sqrt{1-x^2}}{x},\qquad -1 \leqslant x \lt 0 \end{matrix} \right. }[/math]
• [math]\displaystyle{ \arccos x = 2 \arcsin \sqrt \frac{1-x}{2} }[/math]
• [math]\displaystyle{ \arccos x = 2 \arccos \sqrt \frac{1+x}{2} }[/math]
• [math]\displaystyle{ \arccos x = 2 \operatorname{arctg} \sqrt \frac{1-x}{1+x} }[/math]

Получение функции arccos

Дана функция [math]\displaystyle{ y=\cos x }[/math]. На всей своей области определения она является кусочно-монотонной, и, значит, на всей числовой прямой обратное соответствие [math]\displaystyle{ y=\arccos x }[/math] функцией не является. Поэтому рассмотрим отрезок [math]\displaystyle{ [0; \pi] }[/math], на котором функция [math]\displaystyle{ y=\cos x }[/math] строго монотонно убывает и принимает все значения своей области значений только один раз. Тогда на отрезке [math]\displaystyle{ [0; \pi] }[/math] существует обратная функция [math]\displaystyle{ y = \arccos x }[/math], график которой симметричен графику функции [math]\displaystyle{ y=\cos x }[/math] относительно прямой [math]\displaystyle{ y=x }[/math].

Функция arctg

График функции [math]\displaystyle{ y=\operatorname{arctg}\, x }[/math]

Аркта́нгенсом числа x называется такое значение угла [math]\displaystyle{ y, }[/math] выраженное в радианах, для которого [math]\displaystyle{ \operatorname{tg} y = x, \quad -\frac{\pi}{2} \lt y \lt \frac{\pi}{2}. }[/math]

Функция [math]\displaystyle{ y=\operatorname{arctg} x }[/math] определена на всей числовой прямой, всюду непрерывна и ограничена. Она является строго возрастающей.

• [math]\displaystyle{ \operatorname{tg}\,(\operatorname{arctg}\, x)=x }[/math] при [math]\displaystyle{ x \in \mathbb R, }[/math]
• [math]\displaystyle{ \operatorname{arctg}\,(\operatorname{tg}\, y)=y }[/math] при [math]\displaystyle{ -\frac{\pi}{2}\lt y\lt \frac{\pi}{2}, }[/math]
• [math]\displaystyle{ D(\operatorname{arctg}\,x) = (-\infty; \infty) }[/math] (область определения),
• [math]\displaystyle{ E(\operatorname{arctg}\,x) = \left(-\frac{\pi}{2}; \frac{\pi}{2} \right) }[/math] (область значений).

Свойства функции arctg

• [math]\displaystyle{ \operatorname{arctg} (- x) = -\operatorname{arctg} x \qquad }[/math] (функция является нечётной).
• [math]\displaystyle{ \operatorname{arctg} x = \arcsin \frac{x}{\sqrt{1+x^2}}. }[/math]
• [math]\displaystyle{ \operatorname{arctg} x = \left\{\begin{matrix} \arccos \frac{1}{\sqrt{1+x^2}},\qquad x \geqslant 0 \\-\arccos \frac{1}{\sqrt{1+x^2}},\qquad x \leqslant 0\end{matrix}\right. }[/math]
• [math]\displaystyle{ \operatorname{arctg} x = \pi/2-\operatorname{arcctg} x. }[/math]
• [math]\displaystyle{ \operatorname{arctg} x = \left\{\begin{matrix} \operatorname{arcctg} \frac{1}{x},\qquad x \gt 0 \\ \operatorname{arcctg} \frac{1}{x} -\pi,\qquad x \lt 0\end{matrix}\right. }[/math]
• [math]\displaystyle{ \operatorname{arctg} x = -i \operatorname{arth} {ix} }[/math], где [math]\displaystyle{ \operatorname{arth} }[/math] — обратный гиперболический тангенс, ареатангенс.
• [math]\displaystyle{ \operatorname{arth} x = i \operatorname{arctg} {ix}. }[/math]

Получение функции arctg

Дана функция [math]\displaystyle{ y=\operatorname{tg}\, x }[/math]. На всей своей области определения она является кусочно-монотонной, и, значит, обратное соответствие [math]\displaystyle{ y=\operatorname{arctg}\, x }[/math] функцией не является. Поэтому рассмотрим интервал [math]\displaystyle{ (-\pi/2; \pi/2) }[/math], на котором функция [math]\displaystyle{ y=\operatorname{tg}\, x }[/math] строго монотонно возрастает и принимает все значения своей области значений только один раз. Тогда на интервале [math]\displaystyle{ (-\pi/2; \pi/2) }[/math] существует обратная функция [math]\displaystyle{ y=\operatorname{arctg}\, x }[/math], график которой симметричен графику функции [math]\displaystyle{ y=\operatorname{tg}\, x }[/math] относительно прямой [math]\displaystyle{ y=x }[/math].

Функция arcctg

График функции [math]\displaystyle{ y = \operatorname{arcctg} x }[/math]

Арккота́нгенсом числа x называется такое значение угла y (в радианной мере измерения углов), для которого [math]\displaystyle{ \operatorname{ctg}\,y = x,\quad 0 \lt y \lt \pi. }[/math]

Функция [math]\displaystyle{ y=\operatorname{arcctg}\, x }[/math] определена на всей числовой прямой, всюду непрерывна и ограничена. Она является строго убывающей и всюду положительной.

• [math]\displaystyle{ \operatorname{ctg}(\operatorname{arcctg}\, x) = x }[/math] при [math]\displaystyle{ x \in \mathbb R, }[/math]
• [math]\displaystyle{ \operatorname{arcctg}(\operatorname{ctg}\, y) = y }[/math] при [math]\displaystyle{ 0\lt y\lt \pi, }[/math]
• [math]\displaystyle{ D(\operatorname{arcctg} x) = (-\infty; \infty), }[/math]
• [math]\displaystyle{ E(\operatorname{arcctg} x) = (0; \pi). }[/math]

Свойства функции arcctg

• [math]\displaystyle{ \operatorname{arcctg} (-x) = \pi - \operatorname{arcctg} x. }[/math] График функции центрально-симметричен относительно точки [math]\displaystyle{ \left(0; \frac{\pi}{2}\right). }[/math] Функция является индифферентной (ни чётной, ни нечётной).
• [math]\displaystyle{ \operatorname{arcctg} x \gt 0 }[/math] при любых [math]\displaystyle{ x. }[/math]
• [math]\displaystyle{ \operatorname{arcctg} x = \arccos \frac{x}{\sqrt{1+x^2}}. }[/math]
• [math]\displaystyle{ \operatorname{arcctg} x = \left\{\begin{matrix} \arcsin \frac{1}{\sqrt{1+x^2}},\qquad x \geqslant 0 \\\pi-\arcsin \frac{1}{\sqrt{1+x^2}},\qquad x \leqslant 0\end{matrix}\right. }[/math]
• [math]\displaystyle{ \operatorname{arcctg} x = \pi/2-\operatorname{arctg} x. }[/math]
• [math]\displaystyle{ \operatorname{arcctg} x = \left\{\begin{matrix} \operatorname{arctg} \frac{1}{x},\qquad x \gt 0 \\ \operatorname{arctg} \frac{1}{x} +\pi,\qquad x \lt 0\end{matrix}\right. }[/math]

Получение функции arcctg

Дана функция [math]\displaystyle{ y=\operatorname{ctg}\, x }[/math]. На всей своей области определения она является кусочно-монотонной, и, значит, обратное соответствие [math]\displaystyle{ y=\operatorname{arcctg}\, x }[/math] функцией не является. Поэтому рассмотрим интервал [math]\displaystyle{ (0; \pi) }[/math], на котором функция [math]\displaystyle{ y=\operatorname{ctg}\, x }[/math] строго монотонно убывает и принимает все значения своей области значений только один раз. Тогда на интервале [math]\displaystyle{ (0; \pi) }[/math] существует обратная функция [math]\displaystyle{ y=\operatorname{arcctg}\, x }[/math], график которой симметричен графику функции [math]\displaystyle{ y=\operatorname{ctg}\, x }[/math] относительно прямой [math]\displaystyle{ y=x }[/math].

График арккотангенса получается из графика арктангенса, если последний отразить относительно оси ординат (то есть заменить знак аргумента, [math]\displaystyle{ x \rightarrow -x }[/math]) и сместить вверх на π/2; это вытекает из вышеупомянутой формулы [math]\displaystyle{ \operatorname{arcctg} x = \operatorname{arctg} (-x)+\pi/2. }[/math]

Функция arcsec

График функции [math]\displaystyle{ y = \arcsec x }[/math]

Арксе́кансом числа x называется такое значение угла y (в радианной мере измерения углов), для которого [math]\displaystyle{ \sec y = x,\qquad |x| \geqslant 1,\quad 0 \leqslant y \leqslant \pi. }[/math]

Функция [math]\displaystyle{ y=\arcsec x }[/math] непрерывна и ограничена на всей своей области определения. Она является строго возрастающей и всюду неотрицательной.

• [math]\displaystyle{ \sec (\arcsec x)=x }[/math] при [math]\displaystyle{ |x| \geqslant 1, }[/math]
• [math]\displaystyle{ \arcsec (\sec y) = y }[/math] при [math]\displaystyle{ 0 \leqslant y \leqslant \pi. }[/math]
• [math]\displaystyle{ D(\arcsec x)=(-\infty; -1]\cup [1,\infty) }[/math] (область определения),
• [math]\displaystyle{ E(\arcsec x)=[0;\frac{\pi}{2})\cup(\frac{\pi}{2};\pi] }[/math] (область значений).

Свойства функции arcsec

• [math]\displaystyle{ \arcsec(-x) = \pi - \arcsec x. }[/math] График функции центрально-симметричен относительно точки [math]\displaystyle{ \left(0; \frac{\pi}{2}\right). }[/math] Функция является индифферентной (ни чётной, ни нечётной).
• [math]\displaystyle{ \arcsec x \geqslant 0 }[/math] при любых [math]\displaystyle{ x. }[/math]
• [math]\displaystyle{ \arcsec x = \left\{\begin{matrix} \arcsin \frac{\sqrt{x^2-1}}{x},\qquad x \geqslant 1 \\\pi+\arcsin \frac{\sqrt{x^2-1}}{x},\qquad x \leqslant -1\end{matrix}\right. }[/math]
• [math]\displaystyle{ \arcsec x = \frac{\pi}{2}-\operatorname{arccosec} x. }[/math]
• [math]\displaystyle{ \arcsec x = \arccos \frac{1}{x}. }[/math]

Функция arccosec

График функции [math]\displaystyle{ y = \operatorname{arccosec} x }[/math]

Арккосе́кансом числа x называется такое значение угла y (в радианной мере измерения углов), для которого [math]\displaystyle{ \operatorname{cosec} y = x,\qquad |x| \geqslant 1, \quad -\pi/2 \leqslant y \leqslant \pi/2. }[/math]

Функция [math]\displaystyle{ y=\operatorname{arccosec} x }[/math] непрерывна и ограничена на всей своей области определения. Она является строго убывающей.

• [math]\displaystyle{ \operatorname{cosec} (\operatorname{arccosec} x)=x }[/math] при [math]\displaystyle{ |x| \geqslant 1, }[/math]
• [math]\displaystyle{ \operatorname{arccosec} (\operatorname{cosec} y) = y }[/math] при [math]\displaystyle{ -\pi/2 \leqslant y \leqslant \pi/2. }[/math]
• [math]\displaystyle{ D(\operatorname{arccosec} x)=(-\infty; -1]\cup [1,\infty) }[/math] (область определения),
• [math]\displaystyle{ E(\operatorname{arccosec} x)=[-\frac{\pi}{2};0)\cup(0; \frac{\pi}{2}] }[/math] (область значений).

Свойства функции arccosec

• [math]\displaystyle{ \operatorname{arccosec} (-x) = - \operatorname{arccosec} x }[/math] (функция является нечётной).
• [math]\displaystyle{ \operatorname{arccosec}\, x = \operatorname{arctg} \frac{\operatorname{sgn}x}{\sqrt{x^2-1}} = \left\{\begin{matrix} \operatorname{arctg} \frac{1}{\sqrt{x^2-1}},\qquad x \gt 1 \\-\operatorname{arctg} \frac{1}{\sqrt{x^2-1}},\qquad x \lt -1\end{matrix}\right. }[/math]
• [math]\displaystyle{ \operatorname{arccosec} x = \pi/2-\arcsec x. }[/math]
• [math]\displaystyle{ \operatorname{arccosec} x = \arcsin \frac{1}{x}. }[/math]

Разложение в ряды

• [math]\displaystyle{ \displaystyle\arcsin x = x + \frac{x^3}{6} + \frac{3x^5}{40} + \cdots\ = \sum^{\infin}_{n=0} \frac{(2n)!}{4^n (n!)^2 (2n+1)} x^{2n+1} }[/math] для всех [math]\displaystyle{ \left| x \right| \le 1 }[/math]
• [math]\displaystyle{ \displaystyle\arccos x ={\pi\over 2}-\arcsin x={\pi\over 2}- \sum^{\infin}_{n=0} \frac{(2n)!}{4^n (n!)^2 (2n+1)} x^{2n+1} }[/math] для всех [math]\displaystyle{ \left| x \right| \le 1 }[/math]
• [math]\displaystyle{ \displaystyle\operatorname{arctg}\ x = x - \frac{x^3}{3}+ \frac{x^5}{5} - \cdots\ = \sum^{\infin}_{n=1} \frac{(-1)^{n-1}}{2n-1} x^{2n-1} }[/math] для всех [math]\displaystyle{ \left| x \right| \le 1 }[/math]

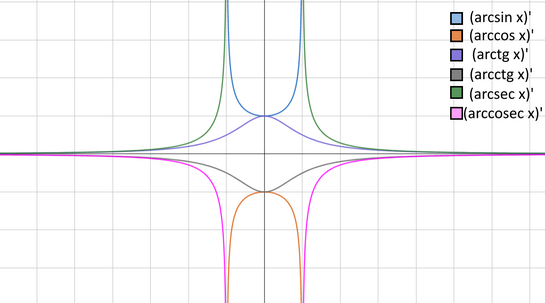
Производные от обратных тригонометрических функций

Все обратные тригонометрические функции бесконечно дифференцируемы в каждой точке своей области определения. Первые производные:

производные обратных тригонометрических функций

Функция [math]\displaystyle{ f(x) }[/math]	Производная [math]\displaystyle{ f'(x) }[/math]	Примечание
[math]\displaystyle{ \arcsin{x} }[/math]	[math]\displaystyle{ \frac{1}{\sqrt{1-x^2}} }[/math]	Доказательство                                  Найти производную арксинуса можно при помощи взаимно обратных функций. [math]\displaystyle{ sin(arcsin((x)) = x }[/math] После чего мы должны взять производную этих обеих функций. [math]\displaystyle{ [sin(arcsin((x))]' = x' }[/math] [math]\displaystyle{ cos(arcsin(x)) \cdot (arcsin(x))' = 1 }[/math] Теперь мы должны выразить производную арксинуса. [math]\displaystyle{ (arcsin(x))' = \frac{1}{cos(arcsin(x))} }[/math] Исходя из тригонометрического тождества([math]\displaystyle{ sin^2 x + cos^2 x = 1 }[/math]) — получаем. [math]\displaystyle{ (arcsin(x))' = \frac{1}{\pm\sqrt{1 - sin^2(arcsin(x))}} }[/math] Для того что-бы понять плюс должен стоять или минус взглянем какие значения. [math]\displaystyle{ D(cos(x)) = [\frac{\pi}{2}; -\frac{\pi}{2}] }[/math] Так как косинус находится в 2-й и 4-й четвертях то, получается что косинус положительный. [math]\displaystyle{ (arcsin(x))' = \frac{1}{\sqrt{1 - sin^2(arcsin(x))}} }[/math] Получается. [math]\displaystyle{ (arcsin(x))' = \frac{1}{\sqrt{1 - x^2}} }[/math]
[math]\displaystyle{ \arccos{x} }[/math]	[math]\displaystyle{ -\frac{1}{\sqrt{1-x^2}} }[/math]	Доказательство                                  Найти производную арккосинуса можно при помощи данного тождества: [math]\displaystyle{ arcsin(x) + arccos(x) = \frac{\pi}{2} }[/math] Теперь находим производную обеих частей этого тождества. [math]\displaystyle{ [arcsin(x) + arccos(x)]' = (\frac{\pi}{2})' }[/math] [math]\displaystyle{ (arcsin(x))' + (arccos(x))' = 0 }[/math] Теперь выражаем производную арккосинуса. [math]\displaystyle{ (arccos(x))' = -(arcsin(x))' }[/math] Получается. [math]\displaystyle{ (arccos(x))' = -\frac{1}{\sqrt{1-x^2}} }[/math]
[math]\displaystyle{ \mathrm{arctg}\ x }[/math]	[math]\displaystyle{ \frac{1}{1+x^2} }[/math]	Доказательство                                  Найти производную арктангенса можно при помощи взаимнообратной функции: [math]\displaystyle{ tg(arctg(x)) = x }[/math] Теперь находим производную обеих частей этого тождества. [math]\displaystyle{ [tg(arctg(x))]' = 1 }[/math] [math]\displaystyle{ \frac{1}{cos^2(arctg(x))} \cdot (arctg(x))' = 1 }[/math] Теперь мы должны выразить производную арктангенса: [math]\displaystyle{ (arctg(x))' = cos^2(arctg(x)) }[/math] Теперь на помощь нам придет на помощь тождество([math]\displaystyle{ cos(x) = \frac{1}{\sqrt{1 + tg^2(x)}} }[/math]): [math]\displaystyle{ (arctg(x))' = (\frac{1}{\sqrt{1 + tg^2(arctg(x))}})^2 }[/math] Получается. [math]\displaystyle{ (arctg(x))' = \frac{1}{1 + x^2} }[/math]
[math]\displaystyle{ \mathrm{arcctg}\ x }[/math]	[math]\displaystyle{ -\frac{1}{1+x^2} }[/math]	Доказательство                                  Найти производную арккотангенса можно при помощи данного тождества: [math]\displaystyle{ arctg(x) + arcctg(x) = \frac{\pi}{2} }[/math] Теперь находим производную обеих частей этого тождества. [math]\displaystyle{ [arctg(x) + arcctg(x)]' = (\frac{\pi}{2})' }[/math] [math]\displaystyle{ (arctg(x))' + (arcctg(x))' = 0 }[/math] Теперь выражаем производную арккотангенса. [math]\displaystyle{ (arcctg(x))' = -(arctg(x))' }[/math] Получается. [math]\displaystyle{ (arcctg(x))' = -\frac{1}{1+x^2} }[/math]
[math]\displaystyle{ \mathrm{arcsec}\ x }[/math]	[math]\displaystyle{ \frac{1}{|x|\sqrt{x^2 - 1}} }[/math]	Доказательство                                  Найти производную арксеканса можно при помощи тождества: [math]\displaystyle{ arcsec(x) = arccos(\frac{1}{x}) }[/math] Теперь находим производную обеих частей этого тождества. [math]\displaystyle{ (arcsec(x))' = (arccos(\frac{1}{x}))' }[/math] [math]\displaystyle{ (arcsec(x))' = -\frac{1}{\sqrt{1 - \frac{1}{x^2}}} \cdot (-\frac{1}{x^2}) }[/math] [math]\displaystyle{ (arcsec(x))' = \frac{1}{x^2\sqrt{\frac{x^2 - 1}{x^2}}} }[/math] [math]\displaystyle{ (arcsec(x))' = \frac{1}{x^2 \frac{\sqrt{x^2 - 1}}{|x|}} }[/math] Получается. [math]\displaystyle{ (arcsec(x))' = \frac{1}{|x| \sqrt{x^2 - 1}} }[/math]
[math]\displaystyle{ \mathrm{arccosec}\ x }[/math]	[math]\displaystyle{ -\frac{1}{|x|\sqrt{x^2 - 1}} }[/math]	Доказательство                                  Найти производную арккосеканса можна при помощи данного тождества: [math]\displaystyle{ arccosec(x) + arcsec(x) = \frac{\pi}{2} }[/math] Теперь находим производную обеих частей этого тождества. [math]\displaystyle{ [arccosec(x) + arcsec(x)]' = (\frac{\pi}{2})' }[/math] [math]\displaystyle{ (arccosec(x))' + (arcsec(x))' = 0 }[/math] Теперь выражаем производную арккосинуса. [math]\displaystyle{ (arccosec(x))' = -(arcsec(x))' }[/math] Получается. [math]\displaystyle{ (arccosec(x))' = -\frac{1}{|x|\sqrt{x^2 - 1}} }[/math]

Интегралы от обратных тригонометрических функций

Неопределённые интегралы

Для действительных и комплексных x:

[math]\displaystyle{ \begin{align} \int \arcsin x\,dx &{}= x\,\arcsin x + \sqrt{1-x^2} + C,\\ \int \arccos x\,dx &{}= x\,\arccos x - \sqrt{1-x^2} + C,\\ \int \operatorname{arctg}\,x\,dx &{}= x\,\operatorname{arctg}\,x - \frac{1}{2}\ln\left(1+x^2\right) + C,\\ \int \operatorname{arcctg}\, x\,dx &{}= x\,\operatorname{arcctg}\, x + \frac{1}{2}\ln\left(1+x^2\right) + C,\\ \int \arcsec x\,dx &{}= x\,\arcsec x - \ln\left(x\left(1+\sqrt{{x^2-1}\over x^2}\,\right)\!\right) + C,\\ \int \operatorname{arccosec}\, x\,dx &{}= x\,\operatorname{arccosec}\, x + \ln\left(x\left(1+\sqrt{{x^2-1}\over x^2}\,\right)\!\right) + C. \end{align} }[/math]

Для действительных x ≥ 1:

[math]\displaystyle{ \begin{align} \int \arcsec x\,dx &{}= x\,\arcsec x - \ln\left(x+\sqrt{x^2-1}\right) + C,\\ \int \operatorname{arccosec}\, x\,dx &{}= x\,\operatorname{arccosec}\, x + \ln\left(x+\sqrt{x^2-1}\right) + C. \end{align} }[/math]

См. также Список интегралов от обратных тригонометрических функций

Использование в геометрии

Прямоугольный треугольник ABC

Обратные тригонометрические функции используются для вычисления углов треугольника, если известны его стороны, например, с помощью теоремы косинусов.

В прямоугольном треугольнике эти функции от отношений сторон сразу дают угол. Так, если катет длины [math]\displaystyle{ a }[/math] является противолежащим для угла [math]\displaystyle{ \alpha }[/math], то

[math]\displaystyle{ \alpha = \arcsin (a/c) = \arccos (b/c) = \operatorname{arctg} (a/b) = \operatorname{arccosec} (c/a) = \arcsec (c/b) = \operatorname{arcctg} (b/a). }[/math]

Связь с натуральным логарифмом

Для вычисления значений обратных тригонометрических функций от комплексного аргумента удобно использовать формулы, выражающие их через натуральный логарифм:

[math]\displaystyle{ \begin{align} \arcsin z & {}= -i \ln (iz+\sqrt{1-z^2})=\frac{\pi}{2}-i\ln (z+\sqrt{z^2-1}), \end{align} }[/math]

[math]\displaystyle{ \begin{align} \arccos z & {}= \dfrac{\pi}{2} + i \ln (iz+\sqrt{1-z^2}), \end{align} }[/math]

[math]\displaystyle{ \begin{align} \operatorname{arctg}\,z & {}= \dfrac{i}{2} ( \ln(1-iz)-\ln(1+iz) ), \end{align} }[/math]

[math]\displaystyle{ \begin{align} \operatorname{arcctg}\,z & {}= \dfrac{i}{2} \left( \ln \left( \dfrac{z-i}{z} \right)-\ln \left( \dfrac{z+i}{z} \right) \right), \end{align} }[/math]

[math]\displaystyle{ \begin{align} \arcsec z & {}= \arccos\left(z^{-1}\right) = \dfrac{\pi}{2} + i \ln \left( \sqrt{1-\dfrac{1}{z^2}} + \dfrac{i}{z} \right), \end{align} }[/math]

[math]\displaystyle{ \begin{align} \operatorname{arccosec}\,z & {}= \arcsin\left(z^{-1}\right) = - i \ln \left( \sqrt{1-\dfrac{1}{z^2}} + \dfrac{i}{z} \right). \end{align} }[/math]

См. также

• Обратные гиперболические функции
• Теорема Данжуа — Лузина

Ссылки

• Weisstein, Eric W. Обратные тригонометрические функции (англ.) на сайте Wolfram MathWorld.
• Математическая энциклопедия / Гл. ред. И. М. Виноградов. — М.: «Советская Энциклопедия», 1982. — [dic.academic.ru/dic.nsf/enc_mathematics/3612/%D0%9E%D0%91%D0%A0%D0%90%D0%A2%D0%9D%D0%AB%D0%95 Т. 3. — с. 1135].
• Обратные тригонометрические функции — статья из Большой советской энциклопедии.  — М.: «Советская Энциклопедия», 1974. — Т. 18. — с. 225.
• Обратные тригонометрические функции // Энциклопедический словарь юного математика / Савин А.П. — М.: Педагогика, 1985. — С. 220—221. — 352 с.
• Построение графиков обратных тригонометрических функций онлайн
• Онлайн калькулятор: обратные тригонометрические функции